Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

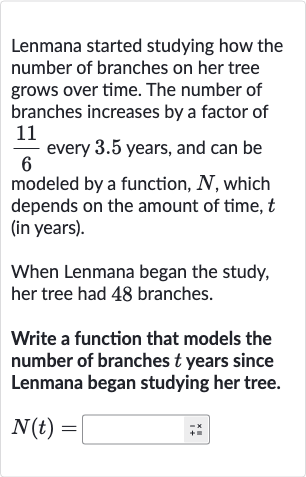
Lenmana started studying how the number of branches on her tree grows over time. The number of branches increases by a factor of
(11)/(6) every 3.5 years, and can be modeled by a function,
N, which depends on the amount of time,
t (in years).
When Lenmana began the study, her tree had 48 branches.
Write a function that models the number of branches
t years since Lenmana began studying her tree.

N(t)=◻

Lenmana started studying how the number of branches on her tree grows over time. The number of branches increases by a factor of $\frac{11}{6}$ every $3$ . $5$ years, and can be modeled by a function, $N$ , which depends on the amount of time, $t$ (in years). $\newline$ When Lenmana began the study, her tree had $48$ branches. $\newline$ Write a function that models the number of branches $t$ years since Lenmana began studying her tree. $\newline$ $N(t)=\square$

View step-by-step help

View step-by-step help

Write exponential functions: word problems

Full solution

Q. Lenmana started studying how the number of branches on her tree grows over time. The number of branches increases by a factor of

\frac{11}{6}

every

3

.

5

years, and can be modeled by a function,

N

, which depends on the amount of time,

t

(in years).

\newline

When Lenmana began the study, her tree had

48

branches.

\newline

Write a function that models the number of branches

t

years since Lenmana began studying her tree.

\newline

N(t)=\square

Define Question Prompt: Let's define the question prompt clearly: ＂Write a function that models the number of branches on Lenmana's tree $t$ years since she began studying it.＂
Identify Initial Number: Identify the initial number of branches $a$ and the growth factor $b$ . $\newline$ Initial number of branches $a$ : $48$ $\newline$ Growth factor $b$ every $3.5$ years: $\frac{11}{6}$
Determine Growth Rate: Determine the growth rate per year by taking the growth factor to the power of $\frac{1}{3.5}$ , since the growth factor is given for every $3.5$ years. $\newline$ Growth rate per year $b$ : $\left(\frac{11}{6}\right)^{\left(\frac{1}{3.5}\right)}$
Write Function $N(t)$ : Write the function $N(t)$ that models the number of branches after $t$ years. $\newline$ $N(t) = a \cdot b^t$ $\newline$ Substitute the values of $a$ and $b$ into the equation. $\newline$ $N(t) = 48 \cdot \left(\frac{11}{6}\right)^{\frac{1}{3.5}}$ ^t
Simplify Function: Simplify the function by combining the terms. $\newline$ $N(t) = 48 \times \left(\frac{11}{6}\right)^{\frac{t}{3.5}}$

More problems from Write exponential functions: word problems

Question

Each year, Francesca earns a salary that is

2 \%

higher than her previous year's salary. In her first

5

years at this job, she earned a total of

\$ 187,345

\newline

What was Francesca's salary in her

1^{\text {st }}

year at this job?

\newline

Round your final answer to the nearest thousand.

\newline

Posted 10 months ago

Question

The following formula gives the temperature's measure in degrees Fahrenheit

F

C

is the measure in degrees Celsius:

\newline

F=\frac{9}{5} C+32

\newline

Rearrange the formula to highlight the measure in degrees Celsius.

\newline

C=

Posted 10 months ago

Question

2010

, the town of Fall River has been experiencing a growth in population.

\newline

The relationship between the elapsed time,

t

, in years, since

2010

and the town's population,

P(t)

, is modeled by the following function.

\newline

P(t)=36,800 \cdot 2^{\frac{t}{25}}

\newline

According to the model, what will the population of Fall River be in

2020

\newline

Round your answer, if necessary, to the nearest whole number.

\newline

Posted 10 months ago

Question

A huge ice glacier in the Himalayas initially covered an area of

45

square kilometers. Because of changing weather patterns, this glacier begins to melt, and the area it covers begins to decrease exponentially.

\newline

The relationship between

A

, the area of the glacier in square kilometers, and

t

, the number of years the glacier has been melting, is modeled by the following equation.

\newline

A=45 e^{-0.05 t}

\newline

How many years will it take for the area of the glacier to decrease to

15

square kilometers?

\newline

Give an exact answer expressed as a natural logarithm.

\newline

Posted 10 months ago

Question

Enzo is studying the black bear population at a large national park.

\newline

He finds that the relationship between the elapsed time

t

, in years, since the beginning of the study, and the black bear population

B(t)

, in the park is modeled by the following function.

\newline

B(t)=2500 \cdot 2^{0.01 t}

\newline

According to the model, what will the black bear population be at that national park in

25

years? Round your answer, if necessary, to the nearest whole number.

\newline

Posted 10 months ago

Question

Find the following trigonometric values.

\newline

Express your answers exactly.

\newline

\begin{array}{l} \cos \left(150^{\circ}\right)= \\ \sin \left(150^{\circ}\right)= \end{array}

Posted 10 months ago

Question

Valeria is playing with her accordion.

\newline

The length of the accordion

A(t)

\mathrm{cm}

) after she starts playing as a function of time

t

(in seconds) can be modeled by a sinusoidal expression of the form

a \cdot \cos (b \cdot t)+d

\newline

t=0

, when she starts playing, the accordion is

15 \mathrm{~cm}

long, which is the shortest it gets.

1

5

seconds later the accordion is at its average length of

21 \mathrm{~cm}

\newline

A(t)

\newline

t

should be in radians.

\newline

A(t)=\square

Posted 10 months ago

Question

On the first day of spring, an entire field of flowering trees blossoms. The population of locusts consuming these flowers rapidly increases as the trees blossom. The locust population gains

87 \%

of its size every

2

4

days, and can be modeled by a function,

L

, which depends on the amount of time,

t

\newline

Before the first day of spring, there were

1100

locusts in the population.

\newline

Write a function that models the locust population

t

days since the first day of spring.

\newline

L(t)=

Posted 10 months ago

Question

Aleksandra started studying how the number of branches on her tree grows over time. Every

1

5

years, the number of branches increases by an addition of

\frac{2}{7}

of the total number of branches. The number of branches can be modeled by a function,

N

, which depends on the amount of time,

t

\newline

When Aleksandra began the study, her tree had

52

\newline

Write a function that models the number of branches

t

years since Aleksandra began studying her tree.

\newline

N(t)=\square

Posted 10 months ago

Question

-14

is an element which loses

\frac{1}{10}

of its mass every

871

years. The mass of a sample of carbon

-14

can be modeled by a function,

M

, which depends on its age,

t

\newline

We measure that the initial mass of a sample of carbon

-14

960

\newline

Write a function that models the mass of the carbon-

14

sample remaining

t

years since the initial measurement.

\newline

M(t)=

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant