Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The following formula gives the temperature's measure in degrees Fahrenheit F, where C is the measure in degrees Celsius: F=(9)/(5)C+32 Rearrange the formula to highlight the measure in degrees Celsius. C=

The following formula gives the temperature's measure in degrees Fahrenheit F F , where C C is the measure in degrees Celsius:\newlineF=95C+32 F=\frac{9}{5} C+32 \newlineRearrange the formula to highlight the measure in degrees Celsius.\newlineC= C=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Write exponential functions: word problemsright chevron icon

Full solution

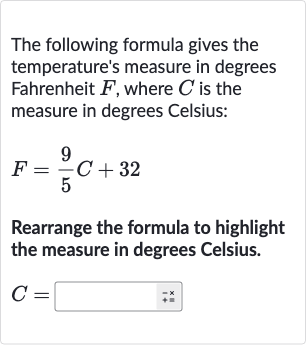
Q. The following formula gives the temperature's measure in degrees Fahrenheit F F , where C C is the measure in degrees Celsius:\newlineF=95C+32 F=\frac{9}{5} C+32 \newlineRearrange the formula to highlight the measure in degrees Celsius.\newlineC= C=
  1. Rephrasing the problem: First, let's rephrase the ＂How can the formula for converting Fahrenheit to Celsius be rearranged to solve for CC, the temperature in degrees Celsius?＂
  2. Subtracting 3232 from both sides: The original formula is F=(95)C+32F = \left(\frac{9}{5}\right)C + 32. To solve for CC, we need to isolate CC on one side of the equation. The first step is to subtract 3232 from both sides of the equation to get rid of the constant term on the right side.\newlineSo, we perform the subtraction: F−32=(95)C+32−32F - 32 = \left(\frac{9}{5}\right)C + 32 - 32.
  3. Equation after subtraction: After subtracting 3232 from both sides, the equation becomes F−32=(95)CF - 32 = \left(\frac{9}{5}\right)C.
  4. Eliminating the fraction: Next, we need to eliminate the fraction (95)(\frac{9}{5}) that is multiplying CC. To do this, we multiply both sides of the equation by the reciprocal of (95)(\frac{9}{5}), which is (59)(\frac{5}{9}).\newlineSo, we perform the multiplication: (59)(F−32)=(59)(95)C(\frac{5}{9})(F - 32) = (\frac{5}{9})(\frac{9}{5})C.
  5. Multiplying by the reciprocal: Multiplying (59)(\frac{5}{9}) by (95)(\frac{9}{5}) on the right side of the equation simplifies to 11, leaving us with CC on the right side.\newlineThe equation now becomes (59)(F−32)=C(\frac{5}{9})(F - 32) = C.
  6. Final rearranged formula: We have successfully isolated CC, and the rearranged formula is C=(59)(F−32)C = \left(\frac{5}{9}\right)(F - 32).

More problems from Write exponential functions: word problems

Question
Each year, Francesca earns a salary that is 2% 2 \% higher than her previous year's salary. In her first 55 years at this job, she earned a total of $187,345 \$ 187,345 .\newlineWhat was Francesca's salary in her 1st  1^{\text {st }} year at this job?\newlineRound your final answer to the nearest thousand.\newlinedollars
Get tutor helpright-arrow

Posted 10 months ago

Question
Since 20102010, the town of Fall River has been experiencing a growth in population.\newlineThe relationship between the elapsed time, t t , in years, since 20102010 and the town's population, P(t) P(t) , is modeled by the following function.\newlineP(t)=36,800⋅2t25 P(t)=36,800 \cdot 2^{\frac{t}{25}} \newlineAccording to the model, what will the population of Fall River be in 20202020 ?\newlineRound your answer, if necessary, to the nearest whole number.\newlinepeople
Get tutor helpright-arrow

Posted 10 months ago

Question
A huge ice glacier in the Himalayas initially covered an area of 4545 square kilometers. Because of changing weather patterns, this glacier begins to melt, and the area it covers begins to decrease exponentially.\newlineThe relationship between A A , the area of the glacier in square kilometers, and t t , the number of years the glacier has been melting, is modeled by the following equation.\newlineA=45e−0.05t A=45 e^{-0.05 t} \newlineHow many years will it take for the area of the glacier to decrease to 1515 square kilometers?\newlineGive an exact answer expressed as a natural logarithm.\newlineyears
Get tutor helpright-arrow

Posted 10 months ago

Question
Enzo is studying the black bear population at a large national park.\newlineHe finds that the relationship between the elapsed time t t , in years, since the beginning of the study, and the black bear population B(t) B(t) , in the park is modeled by the following function.\newlineB(t)=2500⋅20.01t B(t)=2500 \cdot 2^{0.01 t} \newlineAccording to the model, what will the black bear population be at that national park in 2525 years? Round your answer, if necessary, to the nearest whole number.\newlinebears
Get tutor helpright-arrow

Posted 10 months ago

Question
Find the following trigonometric values.\newlineExpress your answers exactly.\newlinecos⁡(150∘)=sin⁡(150∘)= \begin{array}{l} \cos \left(150^{\circ}\right)= \\ \sin \left(150^{\circ}\right)= \end{array}
Get tutor helpright-arrow

Posted 10 months ago

Question
Valeria is playing with her accordion.\newlineThe length of the accordion A(t) A(t) (in cm \mathrm{cm} ) after she starts playing as a function of time t t (in seconds) can be modeled by a sinusoidal expression of the form a⋅cos⁡(b⋅t)+d a \cdot \cos (b \cdot t)+d .\newlineAt t=0 t=0 , when she starts playing, the accordion is 15 cm 15 \mathrm{~cm} long, which is the shortest it gets. 11.55 seconds later the accordion is at its average length of 21 cm 21 \mathrm{~cm} .\newlineFind A(t) A(t) .\newlinet t should be in radians.\newlineA(t)=□ A(t)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
On the first day of spring, an entire field of flowering trees blossoms. The population of locusts consuming these flowers rapidly increases as the trees blossom. The locust population gains 87% 87 \% of its size every 22.44 days, and can be modeled by a function, L L , which depends on the amount of time, t t (in days).\newlineBefore the first day of spring, there were 11001100 locusts in the population.\newlineWrite a function that models the locust population t t days since the first day of spring.\newlineL(t)= L(t)=
Get tutor helpright-arrow

Posted 10 months ago

Question
Aleksandra started studying how the number of branches on her tree grows over time. Every 11.55 years, the number of branches increases by an addition of 27 \frac{2}{7} of the total number of branches. The number of branches can be modeled by a function, N N , which depends on the amount of time, t t (in years).\newlineWhen Aleksandra began the study, her tree had 5252 branches.\newlineWrite a function that models the number of branches t t years since Aleksandra began studying her tree.\newlineN(t)=□ N(t)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
Carbon−14-14 is an element which loses 110 \frac{1}{10} of its mass every 871871 years. The mass of a sample of carbon−14-14 can be modeled by a function, M M , which depends on its age, t t (in years).\newlineWe measure that the initial mass of a sample of carbon−14-14 is 960960 grams.\newlineWrite a function that models the mass of the carbon- 1414 sample remaining t t years since the initial measurement.\newlineM(t)= M(t)=
Get tutor helpright-arrow

Posted 10 months ago

Question
You pick a card at random, put it back, and then pick another card at random.\newline44\newline55\newline66\newline77\newlineWhat is the probability of picking a number greater than 55 and then picking a 44 ?\newlineWrite your answer as a percentage.
Get tutor helpright-arrow

Posted 1 month ago

View all (13)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant