Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

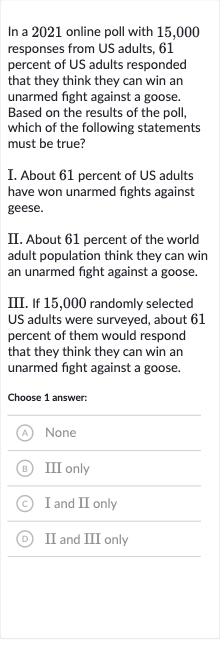
In a 2021 online poll with 15,000 responses from US adults, 61 percent of US adults responded that they think they can win an unarmed fight against a goose. Based on the results of the poll, which of the following statements must be true? I. About 61 percent of US adults have won unarmed fights against geese. II. About 61 percent of the world adult population think they can win an unarmed fight against a goose. III. If 15,000 randomly selected US adults were surveyed, about 61 percent of them would respond that they think they can win an unarmed fight against a goose. Choose 1 answer: (A) None (B) III only (C) I and II only (D) II and III only

In a 20212021 online poll with 1515,000000 responses from US adults, 6161 percent of US adults responded that they think they can win an unarmed fight against a goose. Based on the results of the poll, which of the following statements must be true?\newlineI. About 6161 percent of US adults have won unarmed fights against geese.\newlineII. About 6161 percent of the world adult population think they can win an unarmed fight against a goose.\newlineIII. If 1515,000000 randomly selected US adults were surveyed, about 6161 percent of them would respond that they think they can win an unarmed fight against a goose.\newlineChoose 11 answer:\newline(A) None\newline(B) III only\newline(C) I and II only\newline(D) II and III only

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Interpret confidence intervals for population meansright chevron icon

Full solution

Q. In a 20212021 online poll with 1515,000000 responses from US adults, 6161 percent of US adults responded that they think they can win an unarmed fight against a goose. Based on the results of the poll, which of the following statements must be true?\newlineI. About 6161 percent of US adults have won unarmed fights against geese.\newlineII. About 6161 percent of the world adult population think they can win an unarmed fight against a goose.\newlineIII. If 1515,000000 randomly selected US adults were surveyed, about 6161 percent of them would respond that they think they can win an unarmed fight against a goose.\newlineChoose 11 answer:\newline(A) None\newline(B) III only\newline(C) I and II only\newline(D) II and III only
  1. Calculate US adults: Calculate the number of US adults who think they can win an unarmed fight against a goose using the percentage given.\newline61%61\% of 15,00015,000 = 0.61×15,0000.61 \times 15,000
  2. Perform calculation: Perform the calculation to find the number of US adults. 0.61×15,000=9,1500.61 \times 15,000 = 9,150
  3. Analyze statement I: Analyze statement I: Just because 61%61\% of respondents think they can win doesn't mean they have actually won fights against geese.
  4. Analyze statement II: Analyze statement II: The poll only reflects the opinions of US adults, not the world adult population.
  5. Analyze statement III: Analyze statement III: The statement is a reasonable inference if the poll is representative of the US adult population.
  6. Choose correct answer: Choose the correct answer based on the analysis of the statements.\newlineThe correct answer is (B) IIIIII only.

More problems from Interpret confidence intervals for population means

Question
Based on random samples of river heights, oceanographers estimate that 44,800800 cubic kilometers (km3) \left(\mathrm{km}^{3}\right) of freshwater is discharged into the Arctic Ocean annually. The estimate has a margin of error of 240 km3 240 \mathrm{~km}^{3} at the 90% 90 \% confidence level. Which of the following is the most reasonable claim about the volume of freshwater discharged annually into the Arctic Ocean?\newlineChoose 11 answer:\newlineA) It is between 44,800800 and 5,040 km3 5,040 \mathrm{~km}^{3} .\newlineB) It is between 44,560560 and 5,040 km3 5,040 \mathrm{~km}^{3} .\newline(C) It is between 240240 and 4,800 km3 4,800 \mathrm{~km}^{3} .\newline(D) It is between 240240 and 4,320 km3 4,320 \mathrm{~km}^{3} .
Get tutor helpright-arrow

Posted 10 months ago

Question
A random sample of international flights in 20132013 showed that 79% 79 \% of the flights arrived within 1515 minutes of their scheduled arrival time, and this estimate had a margin of error of 3% 3 \% at a 95% 95 \% confidence level. Which of the following conclusions is best?\newlineChoose 11 answer:\newline(A) Exactly 79% 79 \% arrived within 1515 minutes of their scheduled times.\newline(B) Exactly 76% 76 \% arrived within 1515 minutes of their scheduled times.\newline(C) Between 76% 76 \% and 82% 82 \% arrived within 1515 minutes of their scheduled times.\newline(D) Between 92% 92 \% and 98% 98 \% arrived within 1515 minutes of their scheduled times.
Get tutor helpright-arrow

Posted 10 months ago

Question
A food reporter conducted a survey to determine whether people in a certain city like the new dessert created by Chef Domenica. The reporter asked 8787 people who were waiting in line outside of Chef Domenica's bakery on a Tuesday, and 99 people refused to respond. Which of the following factors makes it least likely that a reliable conclusion can be drawn about whether people in the city like Chef Domenica's new dessert?\newlineChoose 11 answer:\newline(A) Sample size\newline(B) Population size\newline(C) Where the survey was given\newline(D) The number of people who refused to respond
Get tutor helpright-arrow

Posted 9 months ago

Question
The members of a city council wanted to know the opinions of all city residents about collaborating with local farms to set up a farmers market in the city square. The council surveyed a sample of 300300 city residents who have purchased produce from local farms. The survey showed that the majority of those sampled were in favor of the farmers market. Which of the following is true about the city council's survey?\newlineChoose 11 answer:\newlineA It shows that the majority of city residents are in favor of the farmers market.\newline(B) The survey sample is biased because it is not representative of all city residents.\newline(c) The survey sample should have included farmers who live outside the city.\newline(D) The survey should have consisted entirely of farmers.
Get tutor helpright-arrow

Posted 9 months ago

Question
Articles in the American Sociology Review Year\quadTotal number of articles\quadPercent that were quantitative The table summarizes the numbers of articles published in the American Sociology Review in two years. Quantitative articles use numerical data and statistics. How many more quantitative articles were published in the journal in \quad than in \quad? Round to the nearest article.
Get tutor helpright-arrow

Posted 8 months ago

Question
When Famke charges her phone, it gains 22 percentage points each minute. When Famke uses her phone, it loses 55 percentage points each minute.\newlineOnce, Famke used her phone for some time, and then charged it for twice the amount of time she used it. In the end, her phone lost 1010 percentage points.\newlineHow long did Famke use her phone and how long did she charge it?\newlineChoose 11 answer:\newline(A) There is not enough information to determine the exact time Famke used and charged her phone.\newline(B) The given information describes an impossible situation.\newline(C) Famke used her phone for 1010 minutes, and charged her phone for 2020 minutes.\newline(D) Famke used her phone for 2020 minutes, and charged her phone for 1010 minutes.
Get tutor helpright-arrow

Posted 8 months ago

Question
We've updated our Cookie Notice. Please review it now.\newlineCourses\newlineSearch\newlineKhan Academy\newlineDonate \newlineC^(**)\newlineknapke.jace\newlineMy assignments\newlineUnit test\newline66th grade\newlineGraph \newlinex≥−5x \geq -5.\newlineCOURSE: 66TH GRADE > UNIT 77\newlineUnit test\newlineUnit test\newlineEquations & inequalities\newline＂ Math ＂ > ＂ 66th grade ＂ > ＂ Equations \& ＂\newline＂ inequalities ＂ > ＂ Unit test ＂\newlineO 20242024 Khan Academy\newlineTerms ofuse PrivacyPolicy Cookie Notice\newlineHide\newlineDrag the circle to values on the number line.\newlineUse the buttons to switch arrow directions and to make the circle open or filled.\newlineReport a problem
Get tutor helpright-arrow

Posted 8 months ago

Question
It always takes Zach approximately 55 minutes longer to arrive than he says. If Zach says he will arrive in xx minutes, which of the following functions correctly models the approximate number of minutes it actually takes Zach to arrive?
Get tutor helpright-arrow

Posted 8 months ago

Question
One marketing professional recommends that businesses budget between 12%12\% and 16%16\% of their monthly gross sales for rent. If a business earns $540,000\$540,000 in gross sales per year, how many dollars would the adviser recommend as the maximum budget for rent each month?
Get tutor helpright-arrow

Posted 8 months ago

Question
A manager at the Mega Raisin Cereal company wants to confirm that there is an acceptable number of raisins in the company's family-sized boxes. He counted the number of raisins in 100100 randomly sampled boxes from the Mega Raisin factory. From the collected data, he computed a 90%90\% confidence interval of for the mean number of raisins in family-sized boxes of Mega Raisin Cereal.\newlineIs the following conclusion valid?\newlineIf 100100 more samples are taken (with elements chosen randomly and independently), it is expected that exactly 100100 of them will each produce a 90%90\% confidence interval that contains its sample mean.\newlineChoices:\newline(A)yes\newline(B)no\newline
Get tutor helpright-arrow

Posted 7 months ago

View all (40)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant