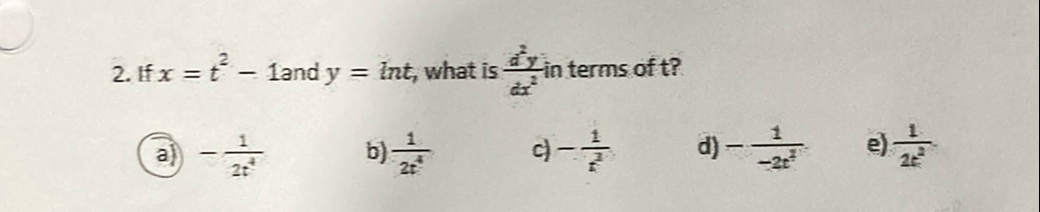If x=t^(2)-1 and y= int, what is (d^(2)y)/(dx^(2)) in terms of t? (ai) -(1)/(2t^(4)) b) (1)/(2t^(4)) c) -(1)/(z^(3)) d) -(1)/(-2e^(2)) e) (1)/(2t^(2))

Question

If  x=t^(2)-1 and  y= int, what is  (d^(2)y)/(dx^(2)) in terms of t? (ai)  -(1)/(2t^(4)) b)  (1)/(2t^(4)) c)  -(1)/(z^(3)) d)  -(1)/(-2e^(2)) e)  (1)/(2t^(2))

Bytelearn · Accepted Answer

Find dy/dx using chain rule: First, we need to find dy/dx using the chain rule since y is an integral with respect to t and x is a function of t. dy/dx = (dy/dt) * (dt/dx)Derivative of y with respect to t: Given y = int, the derivative of y with respect to t is 1, so dy/dt = 1.Find dt/dx: Now, we need to find dt/dx. Since x = t^2 - 1, we can rearrange it to t = sqrt(x + 1). Therefore, dt/dx = 1/(2sqrt(x + 1)).Substitute dt/dx into dy/dx equation: Substitute dt/dx into the dy/dx equation. dy/dx = 1 * (1/(2sqrt(x + 1))) = 1/(2sqrt(x + 1))Find second derivative d^2y/dx^2: Next, we need to find the second derivative, d^2y/dx^2. We'll use the chain rule again, considering that x is a function of t. d^2y/dx^2 = d/dx(dy/dx)Differentiate dy/dx with respect to x: Differentiate dy/dx with respect to x. d^2y/dx^2 = d/dx(1/(2sqrt(x + 1)))Differentiate 1/(2sqrt(x + 1)): To differentiate 1/(2sqrt(x + 1)), we'll use the derivative of the outer function (1/u) with respect to u, which is -1/u^2, and then multiply by the derivative of the inner function (sqrt(x + 1)) with respect to x. d^2y/dx^2 = -1/(4(x + 1)) * (1/(2sqrt(x + 1)))Simplify the expression: Simplify the expression. d^2y/dx^2 = -1/(4(x + 1)(2sqrt(x + 1)))Substitute x back into the equation: Since x = t^2 - 1, substitute x back into the equation. d^2y/dx^2 = -1/(4(t^2)(2t))Simplify the expression further: Simplify the expression further. d^2y/dx^2 = -1/(8t^3)

If $x=t^{2}-1$ and $y =$ $lnt$ , what is $\frac{d^{2}y}{dx^{2}}$ in terms of $t$ ? $\newline$ $a) \frac{-1}{2t^{4}}$ $\newline$ $b) \frac{1}{2t^{4}}$ $\newline$ $c) \frac{-1}{z^{3}}$ $\newline$ $d) \frac{-1}{-2e^{2}}$ $\newline$ $e) \frac{1}{2t^{2}}$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics