AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
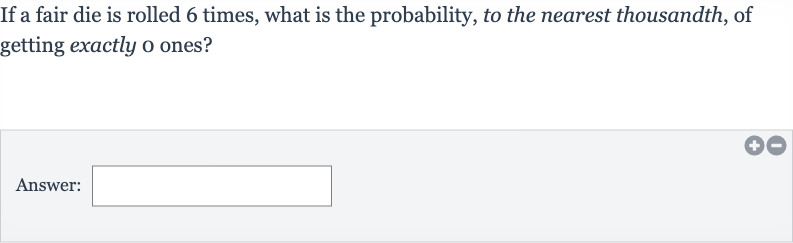
If a fair die is rolled times, what is the probability, to the nearest thousandth, of getting exactly o ones?Answer:
Full solution
Q. If a fair die is rolled times, what is the probability, to the nearest thousandth, of getting exactly o ones?Answer:
- Understand the problem: Understand the problem.We need to calculate the probability of not rolling a one on a six-sided die in each of trials. A fair six-sided die has an equal chance of landing on any of its faces, which means the probability of not rolling a one in a single trial is , since there are other outcomes that are not one.
- Calculate probabilities: Calculate the probability of not rolling a one in each of the trials.Since each roll is independent, we multiply the probability of the event not happening in a single trial by itself times to find the probability of it not happening in trials. This is raised to the power of .
- Perform the calculation: Perform the calculation. when rounded to the nearest thousandth.
- Verify the calculation: Verify the calculation.We can verify the calculation by ensuring that the base and the exponent were correctly applied in the calculation and that the rounding was done to the nearest thousandth.
