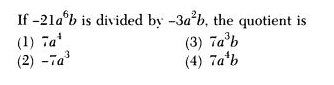AI tutor
Full solution
Q. If is divided , the quotient is() () () ()
- Simplify Expression: Simplify the expression by dividing the terms.
- Check Final Result: Check the final result for correctness. is the quotient when is divided by . The powers of 'a' are subtracted (), and the 'b's cancel out.