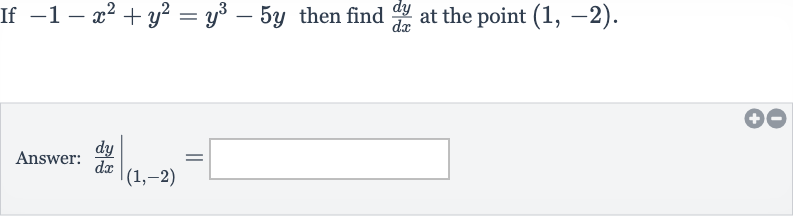
If -1-x^(2)+y^(2)=y^(3)-5y then find (dy)/(dx) at the point (1,-2). Answer: (dy)/(dx)|_((1,-2))=

Question

If  -1-x^(2)+y^(2)=y^(3)-5y then find  (dy)/(dx) at the point  (1,-2). Answer:  (dy)/(dx)|_((1,-2))=

Bytelearn · Accepted Answer

Implicit Differentiation: First, we need to implicitly differentiate both sides of the equation with respect to x. The equation is -1 - x^2 + y^2 = y^3 - 5y. Differentiating both sides with respect to x gives us: (d)/(dx)(-1 - x^2 + y^2) = (d)/(dx)(y^3 - 5y).Differentiate Left Side: Differentiate the left side of the equation term by term. (d)/(dx)(-1) = 0 because the derivative of a constant is 0. (d)/(dx)(-x^2) = -2x because the derivative of x^2 is 2x, and we multiply by the coefficient -1. (d)/(dx)(y^2) = 2y(dy/dx) because y is a function of x, so we use the chain rule. The left side becomes 0 - 2x + 2y(dy/dx).Differentiate Right Side: Differentiate the right side of the equation term by term. (d)/(dx)(y^3) = 3y^2(dy/dx) because we use the chain rule, where the derivative of y^3 is 3y^2, and we multiply by dy/dx. (d)/(dx)(-5y) = -5(dy/dx) because the derivative of 5y with respect to x is 5 times the derivative of y with respect to x. The right side becomes 3y^2(dy/dx) - 5(dy/dx).Combine Derivatives: Combine the derivatives to form an equation: -2x + 2y(dy/dx) = 3y^2(dy/dx) - 5(dy/dx). Now we need to solve for (dy/dx).Group and Factor: Group all the terms containing (dy/dx) on one side and the remaining terms on the other side: 2y(dy/dx) - 3y^2(dy/dx) + 5(dy/dx) = 2x. Factor out (dy/dx) from the left side: (dy/dx)(2y - 3y^2 + 5) = 2x.Solve for dy/dx: Solve for (dy/dx) by dividing both sides by (2y - 3y^2 + 5): (dy/dx) = 2x / (2y - 3y^2 + 5).Substitute Point: Substitute the point (1, -2) into the equation to find (dy/dx) at that point: (dy/dx)|_((1,-2)) = 2(1) / (2(-2) - 3(-2)^2 + 5).Calculate dy/dx: Calculate the value of (dy/dx) at the point (1, -2): (dy/dx)|_((1,-2)) = 2 / (-4 - 3(4) + 5). (dy/dx)|_((1,-2)) = 2 / (-4 - 12 + 5). (dy/dx)|_((1,-2)) = 2 / (-11). (dy/dx)|_((1,-2)) = -2/11.

If $-1-x^{2}+y^{2}=y^{3}-5 y$ then find $\frac{d y}{d x}$ at the point $(1,-2)$ . $\newline$ Answer: $\left.\frac{d y}{d x}\right|_{(1,-2)}=$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

If −1−x2+y2=y3−5y -1-x^{2}+y^{2}=y^{3}-5 y −1−x2+y2=y3−5y then find dydx \frac{d y}{d x} dxdy​ at the point (1,−2) (1,-2) (1,−2).\newlineAnswer: dydx∣(1,−2)= \left.\frac{d y}{d x}\right|_{(1,-2)}= dxdy​∣∣​(1,−2)​=

If $-1-x^{2}+y^{2}=y^{3}-5 y$ then find $\frac{d y}{d x}$ at the point $(1,-2)$ . $\newline$ Answer: $\left.\frac{d y}{d x}\right|_{(1,-2)}=$