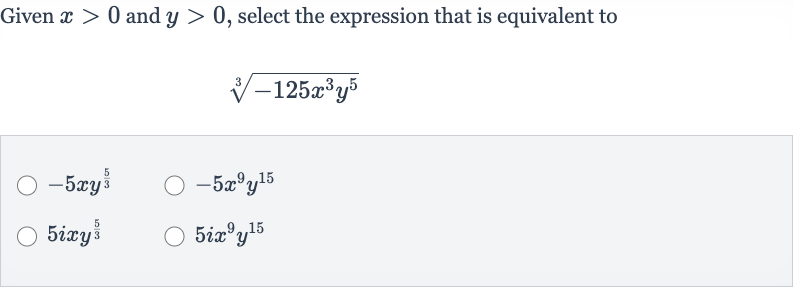Given x 0 and y 0, select the expression that is equivalent to root(3)(-125x^(3)y^(5)) -5xy^((5)/(3)) -5x^(9)y^(15) 5ixy^((5)/(3)) 5ix^(9)y^(15)

Question

Given  x > 0 and  y > 0, select the expression that is equivalent to  root(3)(-125x^(3)y^(5))  -5xy^((5)/(3))  -5x^(9)y^(15)  5ixy^((5)/(3))  5ix^(9)y^(15)

Bytelearn · Accepted Answer

Simplify Constant Term: Simplify the cube root of the constant term. The cube root of -125 is -5 because (-5)^3 = -125.Simplify Variable Term x^(3): Simplify the cube root of the variable term x^(3). The cube root of x^(3) is x because x^(3/3) = x^(1) = x.Simplify Variable Term y^(5): Simplify the cube root of the variable term y^(5). Since the exponent 5 is not a multiple of 3, we cannot take the cube root of y^(5) directly. Instead, we express y^(5) as y^(3) * y^(2) and take the cube root of y^(3), which is y. The remaining y^(2) stays inside the cube root.Combine Results: Combine the results from Steps 1, 2, and 3. Combining the cube roots of the constant and variable terms, we get -5xy times the cube root of y^(2), which can be written as y^(2/3).Write Final Expression: Write the final expression. The final expression is -5xy * y^(2/3), which simplifies to -5xy^(1 + 2/3) = -5xy^(5/3).

Given x>0 and y>0 , select the expression that is equivalent to $\newline$ $\sqrt[3]{-125 x^{3} y^{5}}$ $\newline$ $-5 x y^{\frac{5}{3}}$ $\newline$ $-5 x^{9} y^{15}$ $\newline$ $5 i x y^{\frac{5}{3}}$ $\newline$ $5 i x^{9} y^{15}$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics