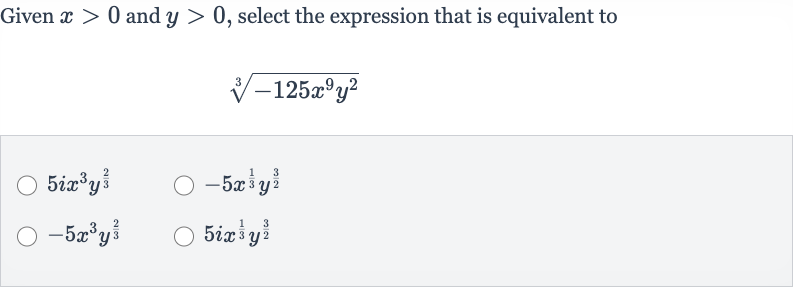Given x 0 and y 0, select the expression that is equivalent to root(3)(-125x^(9)y^(2)) 5ix^(3)y^((2)/(3)) -5x^((1)/(3))y^((3)/(2)) -5x^(3)y^((2)/(3)) 5ix^((1)/(3))y^((3)/(2))

Question

Given  x > 0 and  y > 0, select the expression that is equivalent to  root(3)(-125x^(9)y^(2))  5ix^(3)y^((2)/(3))  -5x^((1)/(3))y^((3)/(2))  -5x^(3)y^((2)/(3))  5ix^((1)/(3))y^((3)/(2))

Bytelearn · Accepted Answer

Simplify -125: Let's first simplify the cube root of the constant and the variables separately. The cube root of -125 is -5 because (-5)^3 = -125.Simplify x^9: Now, let's consider the variable x. The cube root of x^(9) is x^(9/3) because when you take the cube root, you divide the exponent by 3. So, x^(9/3) simplifies to x^3.Simplify y^2: Next, we look at the variable y. The cube root of y^(2) is y^(2/3) because, similarly, you divide the exponent by 3 when taking the cube root.Combine Results: Combining the results from the previous steps, we get the expression -5x^3y^(2/3) as the equivalent expression for the cube root of (-125x^(9)y^(2)).Compare with Options: Now, let's compare our result with the given options to find the matching expression. The correct expression that matches our result is -5x^(3)y^((2)/(3)).

Given x>0 and y>0 , select the expression that is equivalent to $\newline$ $\sqrt[3]{-125 x^{9} y^{2}}$ $\newline$ $5 i x^{3} y^{\frac{2}{3}}$ $\newline$ $-5 x^{\frac{1}{3}} y^{\frac{3}{2}}$ $\newline$ $-5 x^{3} y^{\frac{2}{3}}$ $\newline$ $5 i x^{\frac{1}{3}} y^{\frac{3}{2}}$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics