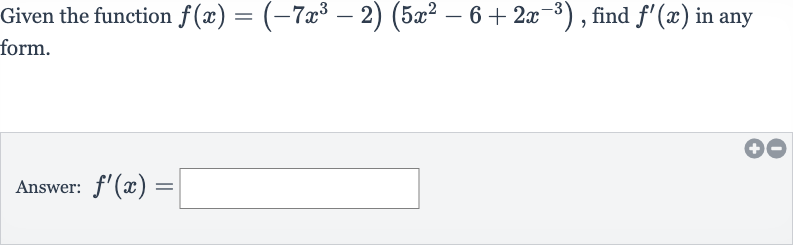
Given the function f(x)=(-7x^(3)-2)(5x^(2)-6+2x^(-3)), find f^(')(x) in any form. Answer: f^(')(x)=

Question

Given the function  f(x)=(-7x^(3)-2)(5x^(2)-6+2x^(-3)), find  f^(')(x) in any form. Answer:  f^(')(x)=

Bytelearn · Accepted Answer

Identify Functions: To find the derivative of the function f(x) = (-7x^3 - 2)(5x^2 - 6 + 2x^(-3)), we will use the product rule. The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.Find u'(x): First, let's identify the two functions that we will be differentiating. We have u(x) = -7x^3 - 2 and v(x) = 5x^2 - 6 + 2x^(-3).Find v'(x): Now, we need to find the derivative of u(x), which is u'(x). The derivative of -7x^3 is -21x^2, and the derivative of a constant is 0. So, u'(x) = -21x^2.Apply Product Rule: Next, we need to find the derivative of v(x), which is v'(x). The derivative of 5x^2 is 10x, the derivative of -6 is 0, and the derivative of 2x^(-3) is -6x^(-4) (using the power rule). So, v'(x) = 10x - 6x^(-4).Expand Terms: Now we can apply the product rule: f'(x) = u'(x)v(x) + u(x)v'(x). Substituting the derivatives we found, we get f'(x) = (-21x^2)(5x^2 - 6 + 2x^(-3)) + (-7x^3 - 2)(10x - 6x^(-4)).Simplify Terms: We will now expand the terms: f'(x) = -21x^2(5x^2) - 21x^2(-6) - 21x^2(2x^(-3)) - 7x^3(10x) - 7x^3(-6x^(-4)) - 2(10x) - 2(-6x^(-4)).Combine Like Terms: Simplify the terms: f'(x) = -105x^4 + 126x^2 - 42x^(-1) - 70x^4 + 42x^(-1) - 20x + 12x^(-4).Combine like terms: f'(x) = (-105x^4 - 70x^4) + 126x^2 - 20x + (42x^(-1) - 42x^(-1)) + 12x^(-4).After combining like terms, we get: f'(x) = -175x^4 + 126x^2 - 20x + 12x^(-4).

Given the function $f(x)=\left(-7 x^{3}-2\right)\left(5 x^{2}-6+2 x^{-3}\right)$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

Given the function f(x)=(−7x3−2)(5x2−6+2x−3) f(x)=\left(-7 x^{3}-2\right)\left(5 x^{2}-6+2 x^{-3}\right) f(x)=(−7x3−2)(5x2−6+2x−3), find f′(x) f^{\prime}(x) f′(x) in any form.\newlineAnswer: f′(x)= f^{\prime}(x)= f′(x)=

Given the function $f(x)=\left(-7 x^{3}-2\right)\left(5 x^{2}-6+2 x^{-3}\right)$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$