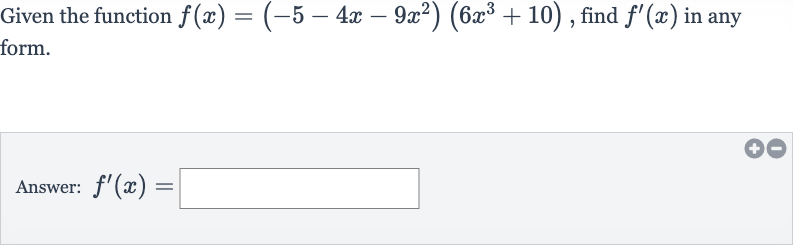
Given the function f(x)=(-5-4x-9x^(2))(6x^(3)+10), find f^(')(x) in any form. Answer: f^(')(x)=

Question

Given the function  f(x)=(-5-4x-9x^(2))(6x^(3)+10), find  f^(')(x) in any form. Answer:  f^(')(x)=

Bytelearn · Accepted Answer

Apply Product Rule: To find the derivative of the function f(x) = (-5 - 4x - 9x^2)(6x^3 + 10), we will use the product rule. The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.Define Functions: Let's denote the first function as u(x) = -5 - 4x - 9x^2 and the second function as v(x) = 6x^3 + 10. We will find the derivatives of u(x) and v(x) separately.Find u'(x): The derivative of u(x) = -5 - 4x - 9x^2 with respect to x is u'(x) = d/dx(-5) - d/dx(4x) - d/dx(9x^2) = 0 - 4 - 18x.Find v'(x): The derivative of v(x) = 6x^3 + 10 with respect to x is v'(x) = d/dx(6x^3) + d/dx(10) = 18x^2 + 0.Use Product Rule Formula: Now we apply the product rule: f'(x) = u'(x)v(x) + u(x)v'(x).Substitute Derivatives: Substitute the derivatives and original functions into the product rule formula: f'(x) = (0 - 4 - 18x)(6x^3 + 10) + (-5 - 4x - 9x^2)(18x^2).Simplify Expression: Simplify the expression by distributing and combining like terms: f'(x) = (-4)(6x^3) + (-4)(10) + (-18x)(6x^3) + (-18x)(10) - 5(18x^2) - 4x(18x^2) - 9x^2(18x^2).Combine Like Terms: Continue simplifying: f'(x) = -24x^3 - 40 - 108x^4 - 180x - 90x^2 - 72x^3 - 162x^4.Final Result: Combine like terms: f'(x) = -108x^4 - 162x^4 - 24x^3 - 72x^3 - 90x^2 - 180x - 40.Finish combining like terms: f'(x) = -270x^4 - 96x^3 - 90x^2 - 180x - 40.

Given the function $f(x)=\left(-5-4 x-9 x^{2}\right)\left(6 x^{3}+10\right)$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

Given the function f(x)=(−5−4x−9x2)(6x3+10) f(x)=\left(-5-4 x-9 x^{2}\right)\left(6 x^{3}+10\right) f(x)=(−5−4x−9x2)(6x3+10), find f′(x) f^{\prime}(x) f′(x) in any form.\newlineAnswer: f′(x)= f^{\prime}(x)= f′(x)=

Given the function $f(x)=\left(-5-4 x-9 x^{2}\right)\left(6 x^{3}+10\right)$ , find $f^{\prime}(x)$ in any form. $\newline$ Answer: $f^{\prime}(x)=$