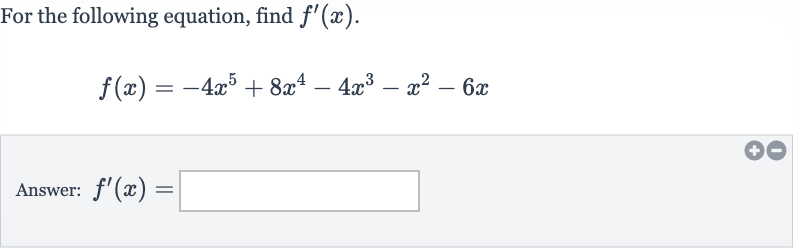
For the following equation, find f^(')(x). f(x)=-4x^(5)+8x^(4)-4x^(3)-x^(2)-6x Answer: f^(')(x)=

Question

For the following equation, find  f^(')(x).  f(x)=-4x^(5)+8x^(4)-4x^(3)-x^(2)-6x Answer:  f^(')(x)=

Bytelearn · Accepted Answer

Apply Power Rule: To find the derivative f^(')(x) of the function f(x) = -4x^(5) + 8x^(4) - 4x^(3) - x^(2) - 6x, we will apply the power rule to each term individually. The power rule states that the derivative of x^n with respect to x is n*x^(n-1).Derivative of -4x^(5): First, we find the derivative of the term -4x^(5). Using the power rule, we get: (d)/(dx)(-4x^(5)) = -4 * 5 * x^(5-1) = -20x^(4).Derivative of 8x^(4): Next, we find the derivative of the term 8x^(4). Using the power rule, we get: (d)/(dx)(8x^(4)) = 8 * 4 * x^(4-1) = 32x^(3).Derivative of -4x^(3): Now, we find the derivative of the term -4x^(3). Using the power rule, we get: (d)/(dx)(-4x^(3)) = -4 * 3 * x^(3-1) = -12x^(2).Derivative of -x^(2): Then, we find the derivative of the term -x^(2). Using the power rule, we get: (d)/(dx)(-x^(2)) = -1 * 2 * x^(2-1) = -2x.Derivative of -6x: Finally, we find the derivative of the term -6x. Since this is a linear term, its derivative is simply the coefficient of x: (d)/(dx)(-6x) = -6.Combine Derivatives: Combining all the derivatives we've calculated, we get the derivative of the entire function f(x): f^(')(x) = -20x^(4) + 32x^(3) - 12x^(2) - 2x - 6.

For the following equation, find $f^{\prime}(x)$ . $\newline$ $f(x)=-4 x^{5}+8 x^{4}-4 x^{3}-x^{2}-6 x$ $\newline$ Answer: $f^{\prime}(x)=$

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

For the following equation, find f′(x) f^{\prime}(x) f′(x).\newlinef(x)=−4x5+8x4−4x3−x2−6x f(x)=-4 x^{5}+8 x^{4}-4 x^{3}-x^{2}-6 x f(x)=−4x5+8x4−4x3−x2−6x\newlineAnswer: f′(x)= f^{\prime}(x)= f′(x)=

For the following equation, find $f^{\prime}(x)$ . $\newline$ $f(x)=-4 x^{5}+8 x^{4}-4 x^{3}-x^{2}-6 x$ $\newline$ Answer: $f^{\prime}(x)=$