AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
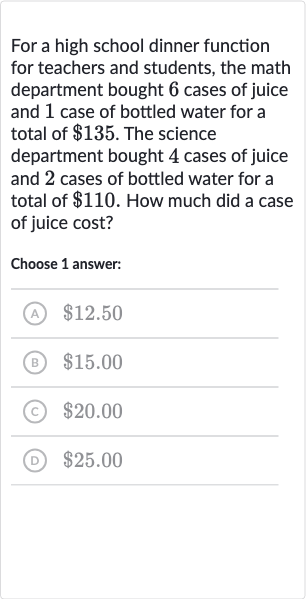
For a high school dinner function for teachers and students, the math department bought cases of juice and case of bottled water for a total of . The science department bought cases of juice and cases of bottled water for a total of . How much did a case of juice cost?Choose answer:(A) (B) (C) (D)
Full solution
Q. For a high school dinner function for teachers and students, the math department bought cases of juice and case of bottled water for a total of . The science department bought cases of juice and cases of bottled water for a total of . How much did a case of juice cost?Choose answer:(A) (B) (C) (D)
- Define Variables: Let's call the cost of a case of juice and the cost of a case of bottled water . We have two equations based on the problem.) )
- Modify Second Equation: First, we can multiply the second equation by to make it easier to eliminate .So,
- Eliminate W: Now, we subtract the modified second equation from the first equation to eliminate W.This simplifies to
- Solve for J: Solving for J, we divide both sides by .
More problems from Solve linear equations with variables on both sides: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
