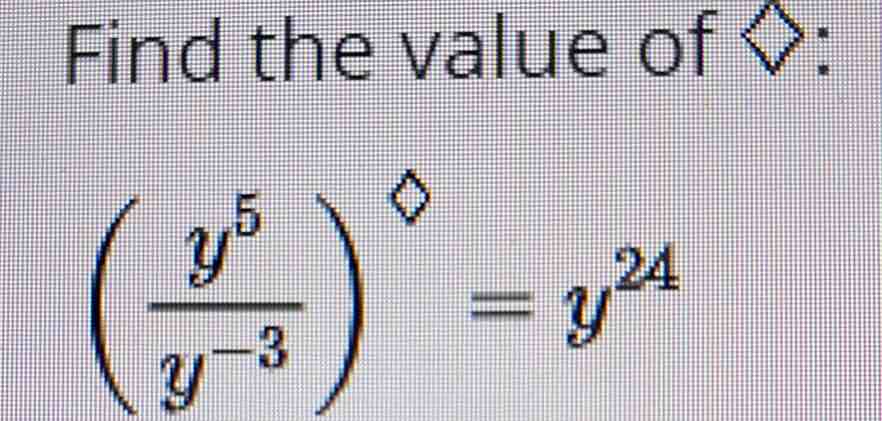AI tutor
Full solution
Q. Find the value of :
- Simplify the Base: First, simplify the base of the equation using the properties of exponents.
- Apply Exponent Rule: Now, we have . According to the properties of exponents, when we raise a power to a power, we multiply the exponents.
- Set Up Equation: To find the value of diamond, we set the exponent of on the left side of the equation equal to the exponent of on the right side of the equation.
- Solve for Diamond: Divide both sides of the equation by to solve for diamond.
- Calculate Final Value: Calculate the value of diamond.