Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the positive solution of the equation. 5x^((7)/(5))-27=10485733 Answer:

Find the positive solution of the equation.\newline5x75−27=10485733 5 x^{\frac{7}{5}}-27=10485733 \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 7right chevron icon
Powers with negative basesright chevron icon

Full solution

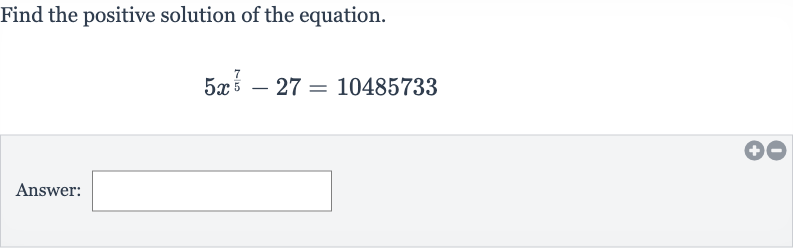
Q. Find the positive solution of the equation.\newline5x75−27=10485733 5 x^{\frac{7}{5}}-27=10485733 \newlineAnswer:
  1. Add 2727 to both sides: Add 2727 to both sides of the equation to isolate the term with the variable on one side.\newline5x75−27+27=10485733+275x^{\frac{7}{5}} - 27 + 27 = 10485733 + 27
  2. Simplify both sides: Simplify both sides of the equation. 5x7/5=104857605x^{7/5} = 10485760
  3. Divide by 55: Divide both sides of the equation by 55 to solve for x75x^{\frac{7}{5}}.\newlinex75=104857605x^{\frac{7}{5}} = \frac{10485760}{5}
  4. Calculate division result: Calculate the division on the right side of the equation. x75=2097152x^{\frac{7}{5}} = 2097152
  5. Raise to power of 55/77: Raise both sides of the equation to the power of 5/75/7 to solve for xx.(x7/5)5/7=20971525/7(x^{7/5})^{5/7} = 2097152^{5/7}
  6. Simplify using exponent property: Simplify the left side of the equation using the property of exponents (am/n)n=am(a^{m/n})^n = a^m.x=20971525/7x = 2097152^{5/7}
  7. Calculate right side: Calculate the right side of the equation to find the value of xx.x≈2097152(5/7)x \approx 2097152^{(5/7)}
  8. Use calculator: Use a calculator to find the value of 20971525/72097152^{5/7}. x≈128x \approx 128

More problems from Powers with negative bases

Question
Write the expression using an exponent.\newline2×22 \times 2\newline______
Get tutor helpright-arrow

Posted 3 months ago

Question
Evaluate the expression. 4−42 4 - 4^2 ____
Get tutor helpright-arrow

Posted 3 months ago

Question
x2=4x^2 = 4
Get tutor helpright-arrow

Posted 2 months ago

Question
x2=9x^2 = 9
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for x. \newlinex2=0x^2 = 0 \newlineWrite your answer in simplified, rationalized form. \newlinex=x = _____ or x=x = _____
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx. \newline8=2x8 = 2^x \newline x=x = _____
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx. \newline6=7x6 = 7^x \newline x=x = _____
Get tutor helpright-arrow

Posted 4 months ago

Question
Select the equivalent expression.\newline((8−5)/(2−2))−4=?((8^{-5})/(2^{-2}))^{-4}=?\newlineChoose 11 answer:\newline(A) \newline(820)/(28)(8^{20})/(2^{8})\newline(B) \newline(26)/(89)(2^{6})/(8^{9})\newline(C) \newline(1)/(8⋅22)(1)/(8\cdot 2^{2})
Get tutor helpright-arrow

Posted 17 days ago

Question
Select the equivalent expression.\newline(b745)−3= ?\left(\frac{b^{7}}{4^{5}}\right)^{-3}=\,?\newlineChoose 11 answer:\newline(A) b21415\frac{b^{21}}{4^{15}}\newline(B) 415b21\frac{4^{15}}{b^{21}}\newline(C) b−21⋅4−15b^{-21}\cdot 4^{-15}
Get tutor helpright-arrow

Posted 2 months ago

Question
Select the equivalent expression.\newline(33⋅66)−3=(3^{3}\cdot6^{6})^{-3}=?\newlineChoose 11 answer:\newline(A) 139⋅618\frac{1}{3^{9}\cdot6^{18}}\newline(B) 61839\frac{6^{18}}{3^{9}}\newline(C) 39618\frac{3^{9}}{6^{18}}
Get tutor helpright-arrow

Posted 2 months ago

View all (79)