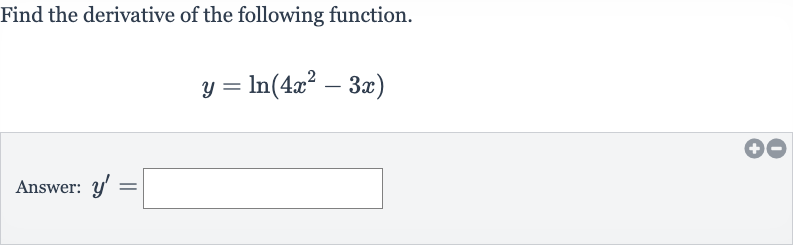
Find the derivative of the following function. y=ln(4x^(2)-3x) Answer: y^(')=

Question

Find the derivative of the following function.  y=ln(4x^(2)-3x) Answer:  y^(')=

Bytelearn · Accepted Answer

Identify Function: Identify the function to differentiate. We have y = ln(4x^2 - 3x). This is a composition of two functions: the natural logarithm function and a quadratic function inside it.Apply Chain Rule: Apply the chain rule for differentiation. The chain rule states that if you have a composite function y = f(g(x)), then the derivative y' is f'(g(x)) * g'(x). Here, f(u) = ln(u) and g(x) = 4x^2 - 3x.Differentiate Outer Function: Differentiate the outer function f(u) = ln(u) with respect to u. The derivative of ln(u) with respect to u is 1/u. So, f'(u) = 1/u.Differentiate Inner Function: Differentiate the inner function g(x) = 4x^2 - 3x with respect to x. The derivative of 4x^2 with respect to x is 8x, and the derivative of -3x with respect to x is -3. So, g'(x) = 8x - 3.Apply Chain Rule with Derivatives: Apply the chain rule using the derivatives from steps 3 and 4. We have f'(u) = 1/u and g'(x) = 8x - 3. Now, we substitute u with g(x) to get f'(g(x)) = 1/(4x^2 - 3x). Then, we multiply f'(g(x)) by g'(x) to get the derivative of y with respect to x. y' = f'(g(x)) * g'(x) = (1/(4x^2 - 3x)) * (8x - 3).Simplify Derivative: Simplify the expression for the derivative. y' = (8x - 3) / (4x^2 - 3x). This is the simplified form of the derivative.

Find the derivative of the following function. $\newline$ $y=\ln \left(4 x^{2}-3 x\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Find derivatives of radical functions

Related topics

Find the derivative of the following function.\newliney=ln⁡(4x2−3x) y=\ln \left(4 x^{2}-3 x\right) y=ln(4x2−3x)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\ln \left(4 x^{2}-3 x\right)$ $\newline$ Answer: $y^{\prime}=$