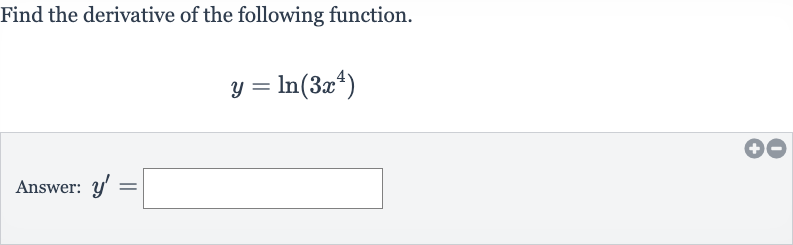
Find the derivative of the following function. y=ln(3x^(4)) Answer: y^(')=

Question

Find the derivative of the following function.  y=ln(3x^(4)) Answer:  y^(')=

Bytelearn · Accepted Answer

Identify function: Identify the function to differentiate. We have y = ln(3x^(4)). We need to find the derivative of this function with respect to x.Apply chain rule: Apply the chain rule for differentiation. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. Here, the outer function is ln(u) and the inner function is u = 3x^(4).Differentiate outer function: Differentiate the outer function. The derivative of ln(u) with respect to u is 1/u. So, if y = ln(u), then y' = 1/u.Differentiate inner function: Differentiate the inner function. The inner function is u = 3x^(4). The derivative of u with respect to x is u' = d/dx(3x^(4)) = 3 * d/dx(x^(4)) = 3 * 4x^(3) = 12x^(3).Apply chain rule: Apply the chain rule. Using the chain rule, the derivative of y with respect to x is y' = (1/u) * (du/dx). Substituting u = 3x^(4) and du/dx = 12x^(3), we get y' = (1/(3x^(4))) * (12x^(3)).Simplify expression: Simplify the expression. Simplify y' by canceling out common factors. The x^(3) in the numerator and denominator cancel out, and we are left with y' = 12/(3x) = 4/x.

Find the derivative of the following function. $\newline$ $y=\ln \left(3 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$

Full solution

More problems from Find derivatives of radical functions

Related topics

Find the derivative of the following function.\newliney=ln⁡(3x4) y=\ln \left(3 x^{4}\right) y=ln(3x4)\newlineAnswer: y′= y^{\prime}= y′=

Find the derivative of the following function. $\newline$ $y=\ln \left(3 x^{4}\right)$ $\newline$ Answer: $y^{\prime}=$