Full solution
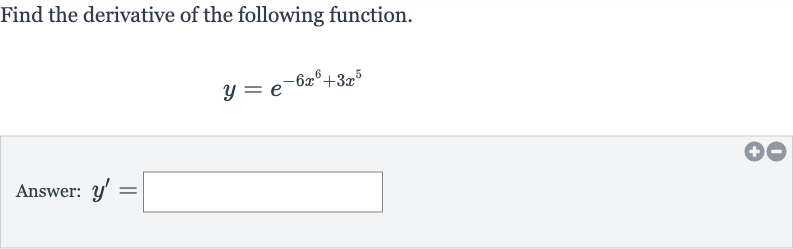
Q. Find the derivative of the following function.Answer:
- Identify function: Identify the function to differentiate.This is an exponential function with a composite function as the exponent.
- Apply chain rule: Apply the chain rule for differentiation, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.Let be the inner function.The outer function is .
- Differentiate outer function: Differentiate the outer function with respect to . If , then .
- Differentiate inner function: Differentiate the inner function with respect to .
- Combine derivatives: Combine the derivatives using the chain rule.Substitute back into the equation.