Full solution
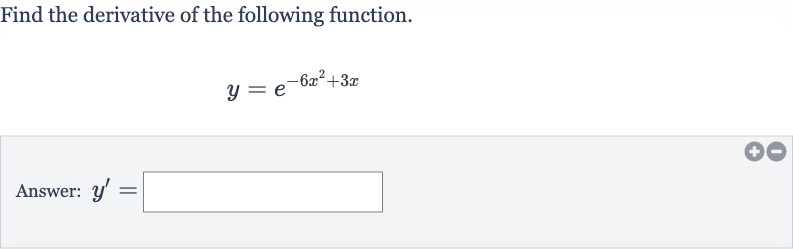
Q. Find the derivative of the following function.Answer:
- Identify function: Identify the function to differentiate.
- Recognize composition: Recognize that this is a composition of functions: the exponential function and a quadratic function. We will use the chain rule to differentiate.The outer function is , where .The inner function is .
- Derivative of outer function: Find the derivative of the outer function with respect to . If , then .
- Derivative of inner function: Find the derivative of the inner function with respect to ..
- Apply chain rule: Apply the chain rule: . .
- Simplify derivative: Simplify the expression for the derivative.