Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the derivative of the following function.

y=3^(-5x^(2))
Answer:
y^(')=

Find the derivative of the following function. $\newline$ $y=3^{-5 x^{2}}$ $\newline$ Answer: $y^{\prime}=$

View step-by-step help

View step-by-step help

Find derivatives of radical functions

Full solution

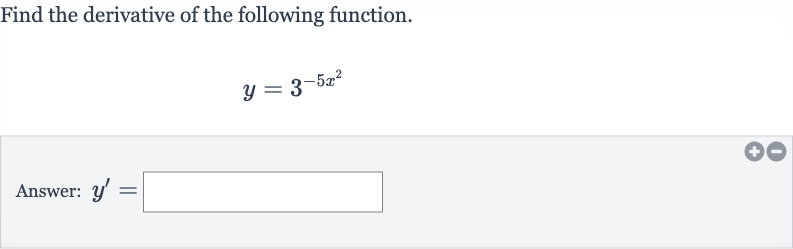
Q. Find the derivative of the following function.

\newline

y=3^{-5 x^{2}}

\newline

Answer:

y^{\prime}=

Identify Functions: We are given the function $y=3^{-5x^{2}}$ . To find the derivative, we will use the chain rule, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.
Derivative of Outer Function: First, let's identify the outer function and the inner function. The outer function is $f(u) = 3^u$ , and the inner function is $u(x) = -5x^2$ . We will need to take the derivative of both of these functions.
Derivative of Inner Function: The derivative of the outer function $f(u) = 3^u$ with respect to $u$ is $f'(u) = 3^u \cdot \ln(3)$ , using the fact that the derivative of $a^x$ with respect to $x$ is $a^x \cdot \ln(a)$ .
Apply Chain Rule: The derivative of the inner function $u(x) = -5x^2$ with respect to $x$ is $u'(x) = \frac{d}{dx}(-5x^2) = -10x$ .
Substitute Derivatives: Now we apply the chain rule. The derivative of $y$ with respect to $x$ is $y' = f'(u) \cdot u'(x)$ . Substituting the derivatives we found, we get $y' = (3^{-5x^2} \cdot \ln(3)) \cdot (-10x)$ .
Simplify Final Derivative: Simplify the expression to get the final derivative. $y' = -10x \cdot \ln(3) \cdot 3^{-5x^2}$ .

More problems from Find derivatives of radical functions

Question

Find the derivative of

f(x)

\newline

f(x) = \cos(x)

\newline

f'(x) =

Posted 1 month ago

Question

Find the derivative of

f(x)

\newline

f(x) = \tan(x)

\newline

f'(x) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = e^x

\newline

f'\left(x\right) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = \ln(x)

\newline

f'(x) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = \tan^{-1}{x}

\newline

f'(x) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = x e^x

\newline

f'\left(x\right) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = \frac{e^x}{x}

\newline

f'(x) =

Posted 2 months ago

Question

Find the derivative of

f(x)

\newline

f(x) = e^{(x + 1)}

\newline

f'\left(x\right) =

Posted 1 month ago

Question

Find the derivative of

f(x)

\newline

f(x) = \sqrt{x+3}

\newline

f'(x) =

Posted 2 months ago

Question

Find the derivative of

g(x)

\newline

g(x) = \ln(2x)

\newline

g^{\prime}(x) =

Posted 3 months ago