AI tutor
Full solution
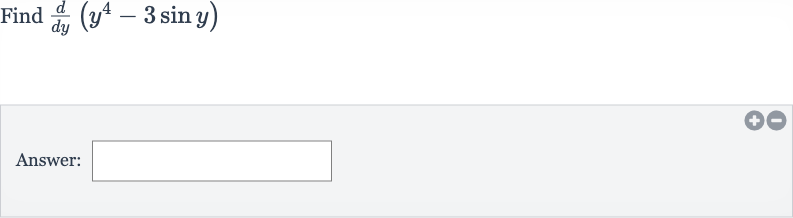
Q. Find Answer:
- Apply Power Rule: Differentiate the function with respect to . We will apply the power rule to and the derivative of the sine function to .
- Power Rule for : Apply the power rule to .The power rule states that .
- Differentiate : Differentiate with respect to . The derivative of with respect to is , and we need to multiply this by the constant .
- Combine Results: Combine the results from Step and Step .