AI tutor
Full solution
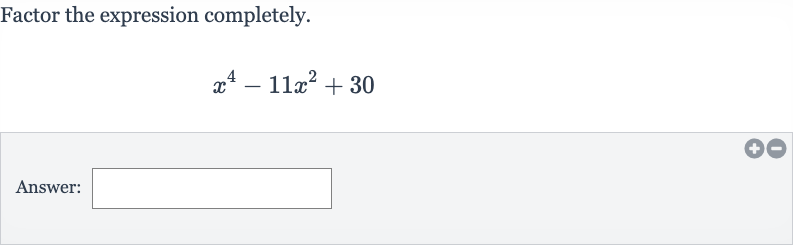
Q. Factor the expression completely.Answer:
- Recognize Quadratic Form: Recognize the expression as a quadratic in form.The given expression is a quadratic in form, where is the variable instead of . We can substitute to make it look like a standard quadratic equation: .
- Factor Quadratic Equation: Factor the quadratic equation.We need to find two numbers that multiply to and add up to . These numbers are and . So we can write the quadratic equation as .
- Substitute Back : Substitute back for . Now we replace with to get the factors in terms of : .
- Check Further Factorization: Check for further factorization.Both and are difference of squares, but neither nor are perfect squares, so they cannot be factored further using real numbers. Therefore, the expression is fully factored.