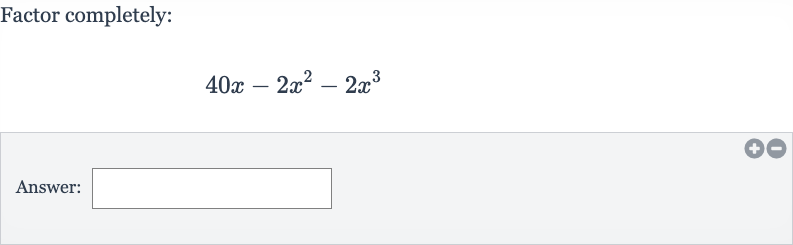Factor completely: 40 x-2x^(2)-2x^(3) Answer:

Question

Factor completely:  40 x-2x^(2)-2x^(3) Answer:

Bytelearn · Accepted Answer

Identify Common Factor: First, we identify the common factor in all terms of the polynomial 40x - 2x^2 - 2x^3. The common factor is 2x, as it is the greatest common factor that can be divided evenly into each term.Factor Out Common Factor: Next, we factor out the common factor 2x from each term of the polynomial. 2x(20 - x - x^2)Rearrange Terms: We notice that the order of the terms inside the parentheses is not standard. We should rearrange the terms in descending order of the powers of x. 2x(-x^2 - x + 20)Factor Quadratic Expression: Now, we look to factor the quadratic expression inside the parentheses. We need two numbers that multiply to -20 (the constant term) and add up to -1 (the coefficient of the middle term). The numbers that satisfy these conditions are -5 and 4.Split Middle Term: We factor the quadratic expression by splitting the middle term using the numbers -5 and 4. 2x(-x^2 - 5x + 4x + 20)Factor by Grouping: Next, we group the terms in pairs and factor by grouping. 2x[(-x^2 - 5x) + (4x + 20)]Factor Out Common Factor: We factor out the common factor from each group. 2x[-x(x + 5) + 4(x + 5)]Factor Out Common Factor: We notice that (x + 5) is a common factor in both groups, so we factor it out. 2x(x + 5)(-x + 4)Write Completely Factored Form: Finally, we write the completely factored form of the polynomial, ensuring that all signs are correct. 2x(x + 5)(-x + 4) is the completely factored form of the polynomial.

Factor completely: $\newline$ $40 x-2 x^{2}-2 x^{3}$ $\newline$ Answer:

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

Factor completely:\newline40x−2x2−2x3 40 x-2 x^{2}-2 x^{3} 40x−2x2−2x3\newlineAnswer:

Factor completely: $\newline$ $40 x-2 x^{2}-2 x^{3}$ $\newline$ Answer: