AI tutor
Full solution
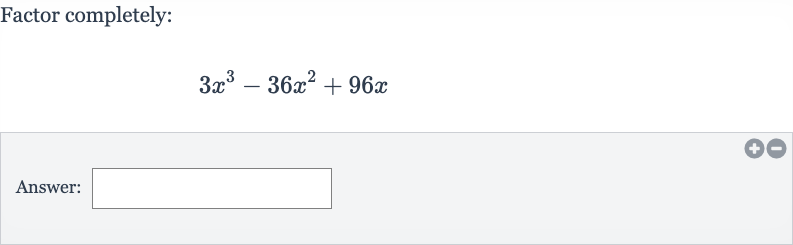
Q. Factor completely:Answer:
- Identify Common Factors: Identify common factors in all terms.The polynomial is . We can see that each term has a common factor of . We will factor out from each term.Calculation:
- Factor Quadratic Expression: Factor the quadratic expression.We now have the quadratic expression , which we need to factor. We are looking for two numbers that multiply to and add up to .Calculation: The numbers are and because and .
- Write Factored Form: Write the factored form of the quadratic expression.Using the numbers found in Step , we can write the quadratic expression as .Calculation:
- Combine Factored Quadratic: Combine the factored quadratic with the common factor factored out in Step .We now multiply the common factor by the factored quadratic expression to get the completely factored form of the original polynomial.Calculation: