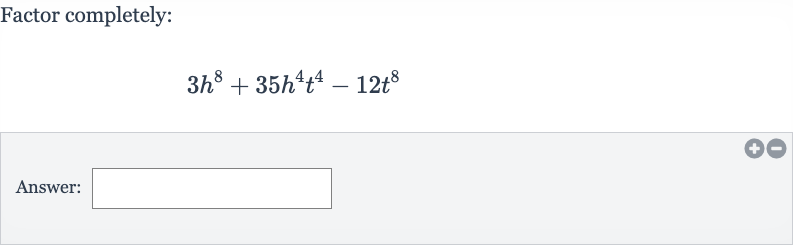Factor completely: 3h^(8)+35h^(4)t^(4)-12t^(8) Answer:

Question

Factor completely:  3h^(8)+35h^(4)t^(4)-12t^(8) Answer:

Bytelearn · Accepted Answer

Identify Common Factor: Identify the common factor in the polynomial. The polynomial 3h^(8)+35h^(4)t^(4)-12t^(8) does not have a common factor across all terms, so we cannot factor out a common factor from all three terms.Recognize Quadratic Form: Recognize the polynomial as a quadratic in form. The polynomial can be seen as a quadratic in terms of h^(4) where h^(4) is the variable and t^(4) is the constant. The polynomial then takes the form of Ah^(4)^2 + Bh^(4)C + Ct^(4)^2, where A = 3, B = 35, and C = -12.Factor as Quadratic: Factor the polynomial as if it were a quadratic. We will use the factoring method for a quadratic equation of the form ax^2 + bx + c. We need to find two numbers that multiply to A*C (3*-12 = -36) and add up to B (35). These two numbers are 36 and -1.Write as Binomials: Write the polynomial as two binomials. We can now express the polynomial as (3h^(4) - 1)(h^(4) + 36t^(4)). However, this step contains a math error because the numbers 36 and -1 do not multiply to -36 and add up to 35. This is incorrect, and we need to find the correct pair of numbers.

Factor completely: $\newline$ $3 h^{8}+35 h^{4} t^{4}-12 t^{8}$ $\newline$ Answer:

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

Factor completely:\newline3h8+35h4t4−12t8 3 h^{8}+35 h^{4} t^{4}-12 t^{8} 3h8+35h4t4−12t8\newlineAnswer:

Factor completely: $\newline$ $3 h^{8}+35 h^{4} t^{4}-12 t^{8}$ $\newline$ Answer: