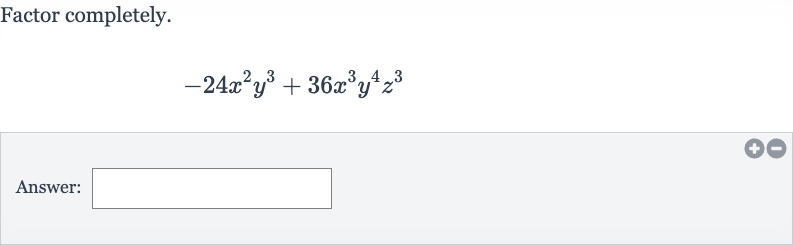AI tutor
Full solution
Q. Factor completely.Answer:
- Identify GCF: Identify the greatest common factor (GCF) of the terms and . The GCF of the coefficients ( and ) is . The GCF of the terms ( and ) is . The GCF of the terms ( and ) is . There is no term in the first expression, so is not part of the GCF. The GCF is .
- Factor out GCF: Factor out the GCF from each term in the expression..
- Check for Simplification: Check if the factored expression can be simplified further.The expression inside the parentheses cannot be factored further since there is no common factor between the terms.
- Write Final Expression: Write down the final factored expression.The completely factored form of the expression is .