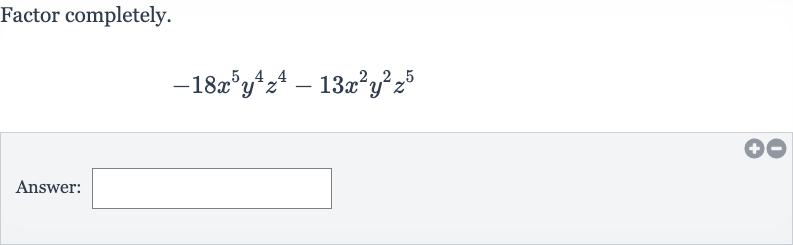AI tutor
Full solution
Q. Factor completely.Answer:
- Identify GCF: Identify the greatest common factor (GCF) of the terms in the expression. and share a common factor of .
- Factor out GCF: Factor out the GCF from each term in the expression.
- Simplify expression: Simplify the expression inside the parentheses.So, the factored expression is
- Combine terms: Combine the GCF and the simplified terms to write the final factored expression.The completely factored form is .