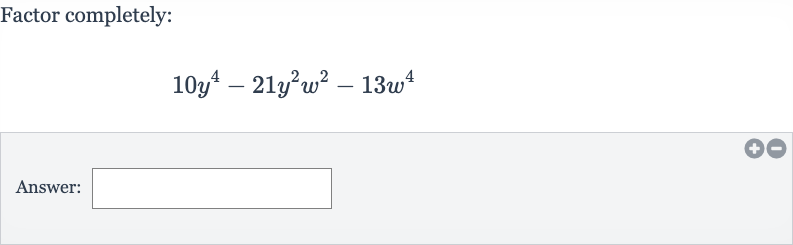
Factor completely: 10y^(4)-21y^(2)w^(2)-13w^(4) Answer:

Question

Factor completely:  10y^(4)-21y^(2)w^(2)-13w^(4) Answer:

Bytelearn · Accepted Answer

Recognize as quadratic in y^2: Recognize the polynomial as a quadratic in terms of y^2. The given polynomial 10y^(4)-21y^(2)w^(2)-13w^(4) can be treated as a quadratic equation in y^2, where y^2 is the variable and 10, -21w^2, and -13w^4 are the coefficients.Factor using middle term: Factor the quadratic polynomial using the middle term factor method or any other suitable factoring technique. We need to find two numbers that multiply to (10)(-13w^4) = -130w^4 and add up to -21w^2. These two numbers are -26w^2 and 5w^2.Rewrite middle term: Rewrite the middle term of the polynomial using the two numbers found in Step 2. 10y^(4)-26y^(2)w^(2)+5y^(2)w^(2)-13w^(4)Factor by grouping: Factor by grouping. Group the terms to factor by grouping: (10y^(4)-26y^(2)w^(2)) + (5y^(2)w^(2)-13w^(4)) Now factor out the common factors from each group: 2y^2(5y^2-13w^2) + w^2(5y^2-13w^2)Factor out common factor: Factor out the common binomial factor. Both groups contain the common factor (5y^2-13w^2), so we can factor this out: (2y^2 + w^2)(5y^2 - 13w^2)Check for further factorization: Check the factors to ensure they cannot be factored further. The factors (2y^2 + w^2) and (5y^2 - 13w^2) cannot be factored further over the integers. Therefore, the factorization is complete.

Factor completely: $\newline$ $10 y^{4}-21 y^{2} w^{2}-13 w^{4}$ $\newline$ Answer:

Full solution

More problems from Find derivatives of using multiple formulae

Related topics

Factor completely:\newline10y4−21y2w2−13w4 10 y^{4}-21 y^{2} w^{2}-13 w^{4} 10y4−21y2w2−13w4\newlineAnswer:

Factor completely: $\newline$ $10 y^{4}-21 y^{2} w^{2}-13 w^{4}$ $\newline$ Answer: