Full solution
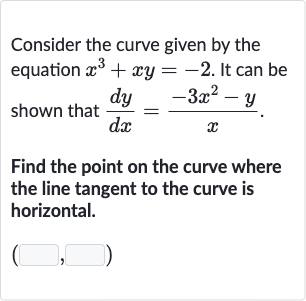
Q. Consider the curve given by the equation . It can be shown that .Find the point on the curve where the line tangent to the curve is horizontal. ,
- Set Derivative Equal to Zero: To find where the tangent line to the curve is horizontal, we need to set the derivative equal to zero, because a horizontal line has a slope of .
- Solve for y: Since the numerator of the derivative must be zero for the derivative to be zero, we set the numerator equal to zero and solve for y.
- Substitute back: Now we substitute back into the original equation of the curve to find the corresponding -value.
- Solve for x: Divide both sides by to solve for .
- Find y-value: Take the cube root of both sides to solve for .
- Final Point: Now that we have the -value, we can find the corresponding -value using the equation .
- Final Point: Now that we have the -value, we can find the corresponding -value using the equation .We have found the point on the curve where the tangent line is horizontal. The point is .
More problems from Power rule
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help