AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
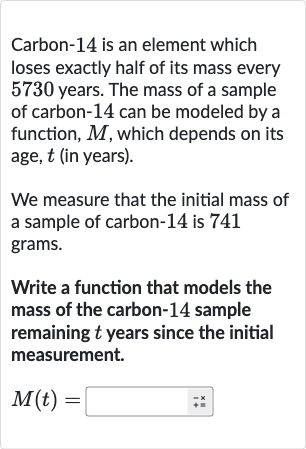
Carbon is an element which loses exactly half of its mass every years. The mass of a sample of carbon- can be modeled by a function, , which depends on its age, (in years).We measure that the initial mass of a sample of carbon is grams.Write a function that models the mass of the carbon- sample remaining years since the initial measurement.
Full solution
Q. Carbon is an element which loses exactly half of its mass every years. The mass of a sample of carbon- can be modeled by a function, , which depends on its age, (in years).We measure that the initial mass of a sample of carbon is grams.Write a function that models the mass of the carbon- sample remaining years since the initial measurement.
- Identify initial mass and decay factor: Identify the initial mass () and the decay factor ().The initial mass of the carbon sample is given as grams. This is the value of '' in our exponential decay function.Since the mass of carbon loses half of its mass every years, the decay factor '' is (because every years, the mass is multiplied by ).
- Determine decay rate per year: Determine the decay rate per year.The decay rate per year is not directly given, but we know that the mass halves every years. Therefore, the decay factor '' is raised to the power of , where is the number of years since the initial measurement.
- Write exponential decay function: Write the exponential decay function.The general form of an exponential decay function is . In this case, is the initial mass, and is the decay factor raised to the power of . Therefore, the function that models the mass of the carbon sample remaining years since the initial measurement is:
More problems from Write exponential functions: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
