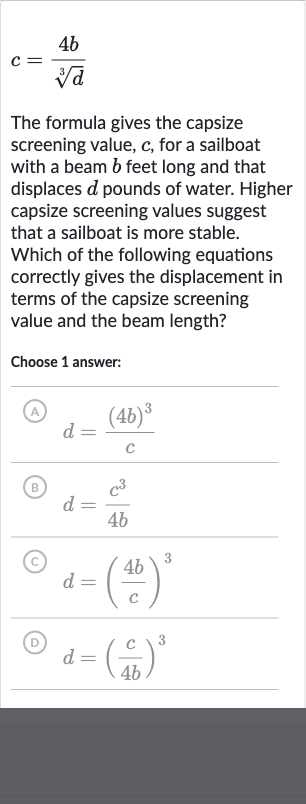
c=(4b)/(root(3)(d)) The formula gives the capsize screening value, c, for a sailboat with a beam b feet long and that displaces d pounds of water. Higher capsize screening values suggest that a sailboat is more stable. Which of the following equations correctly gives the displacement in terms of the capsize screening value and the beam length? Choose 1 answer: (A) d=((4b)^(3))/(c) (B) d=(c^(3))/(4b) (C) d=((4b)/(c))^(3) (D) d=((c)/(4b))^(3)

Question

c=(4b)/(root(3)(d)) The formula gives the capsize screening value,  c, for a sailboat with a beam  b feet long and that displaces  d pounds of water. Higher capsize screening values suggest that a sailboat is more stable. Which of the following equations correctly gives the displacement in terms of the capsize screening value and the beam length? Choose 1 answer: (A)  d=((4b)^(3))/(c) (B)  d=(c^(3))/(4b) (C)  d=((4b)/(c))^(3) (D)  d=((c)/(4b))^(3)

Bytelearn · Accepted Answer

Given Equation: The original equation is given by c = (4b) / (root(3)(d)). We want to solve for d in terms of c and b.Isolate Term with d: First, we will isolate the term containing d on one side of the equation by multiplying both sides by (root(3)(d)). This gives us c * (root(3)(d)) = 4b.Divide by c: Next, we divide both sides of the equation by c to get (root(3)(d)) = (4b) / c.Get Rid of Cube Root: To solve for d, we need to get rid of the cube root. We do this by raising both sides of the equation to the power of 3, which gives us d = ((4b) / c)^3.Final Equation Comparison: Comparing the result with the given options, we find that the correct equation is (C) d = ((4b) / c)^3.

Full solution

More problems from Find derivatives of using multiple formulae

Related topics