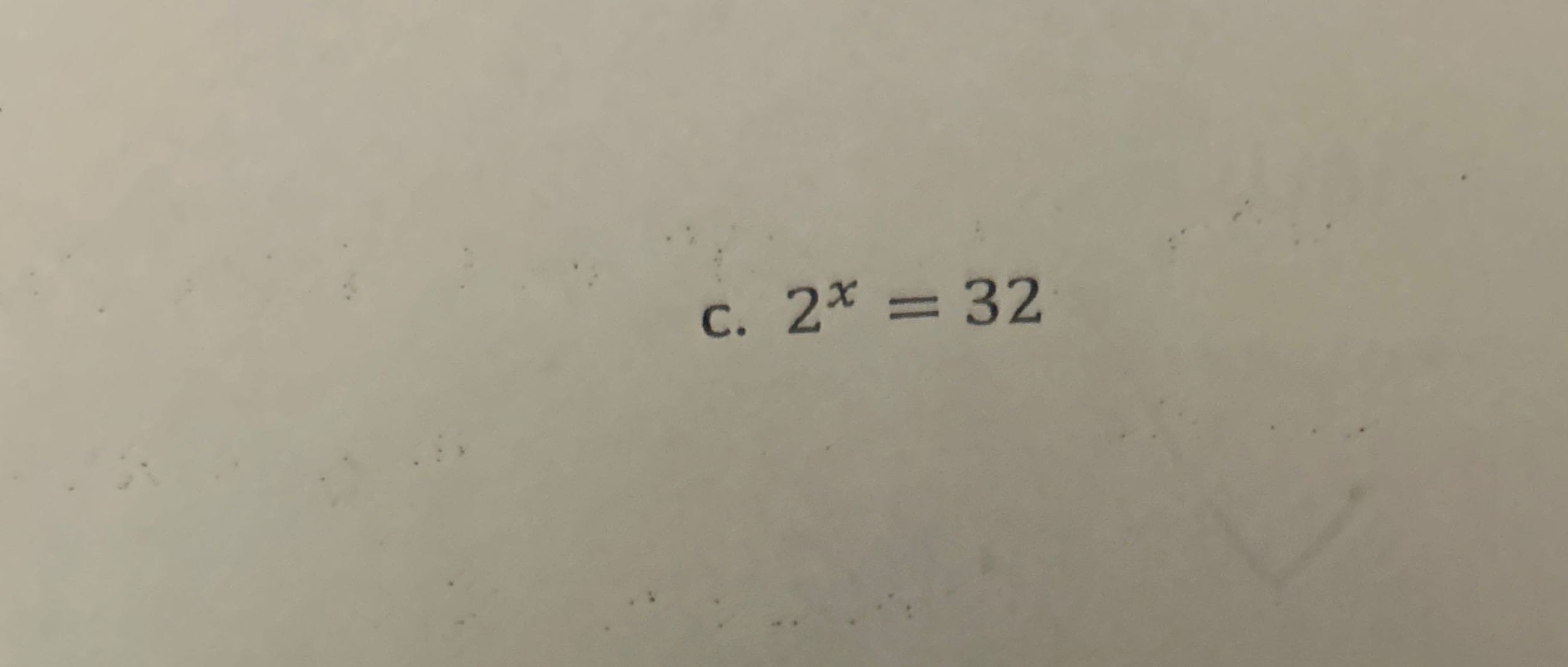Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for
x:
2^(x)=32

Solve for $x$ : $2^{x}=32$

View step-by-step help

View step-by-step help

Solve equations with variable exponents

Full solution

Q. Solve for

x

:

2^{x}=32

Identify base and target: Identify the base and the target value. $2$ raised to some power equals $32$ . We need to find that power. $\newline$ Base: $2$ $\newline$ Target Value: $32$
Express as power of $2$ : Express $32$ as a power of $2$ . $\newline$ $32$ can be written as $2^5$ because $2 \times 2 \times 2 \times 2 \times 2 = 32$ .
Set equation and solve: Set the equation and solve for $x$ . $\newline$ Since $2^x = 32$ and $32 = 2^5$ , then $2^x = 2^5$ . $\newline$ Therefore, $x = 5$ .

More problems from Solve equations with variable exponents

Question

Write the expression using an exponent.

\newline

2 \times 2

\newline

Posted 8 months ago

Question

Evaluate the expression.

4 - 4^2

Posted 8 months ago

Question

x^2 = 4

Posted 7 months ago

Question

x^2 = 9

Posted 9 months ago

Question

\newline

x^2 = 0

\newline

Write your answer in simplified, rationalized form.

\newline

x =

x =

Posted 9 months ago

Question

x

\newline

8 = 2^x

\newline

x =

Posted 9 months ago

Question

x

\newline

6 = 7^x

\newline

x =

Posted 9 months ago

Question

Select the equivalent expression.

\newline

((8^{-5})/(2^{-2}))^{-4}=?

\newline

1

\newline

\newline

(8^{20})/(2^{8})

\newline

\newline

(2^{6})/(8^{9})

\newline

\newline

(1)/(8\cdot 2^{2})

Posted 6 months ago

Question

Select the equivalent expression.

\newline

\left(\frac{b^{7}}{4^{5}}\right)^{-3}=\,?

\newline

1

\newline

\frac{b^{21}}{4^{15}}

\newline

\frac{4^{15}}{b^{21}}

\newline

b^{-21}\cdot 4^{-15}

Posted 7 months ago

Question

Select the equivalent expression.

\newline

(3^{3}\cdot6^{6})^{-3}=

\newline

1

\newline

\frac{1}{3^{9}\cdot6^{18}}

\newline

\frac{6^{18}}{3^{9}}

\newline

\frac{3^{9}}{6^{18}}

Posted 8 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant