Full solution
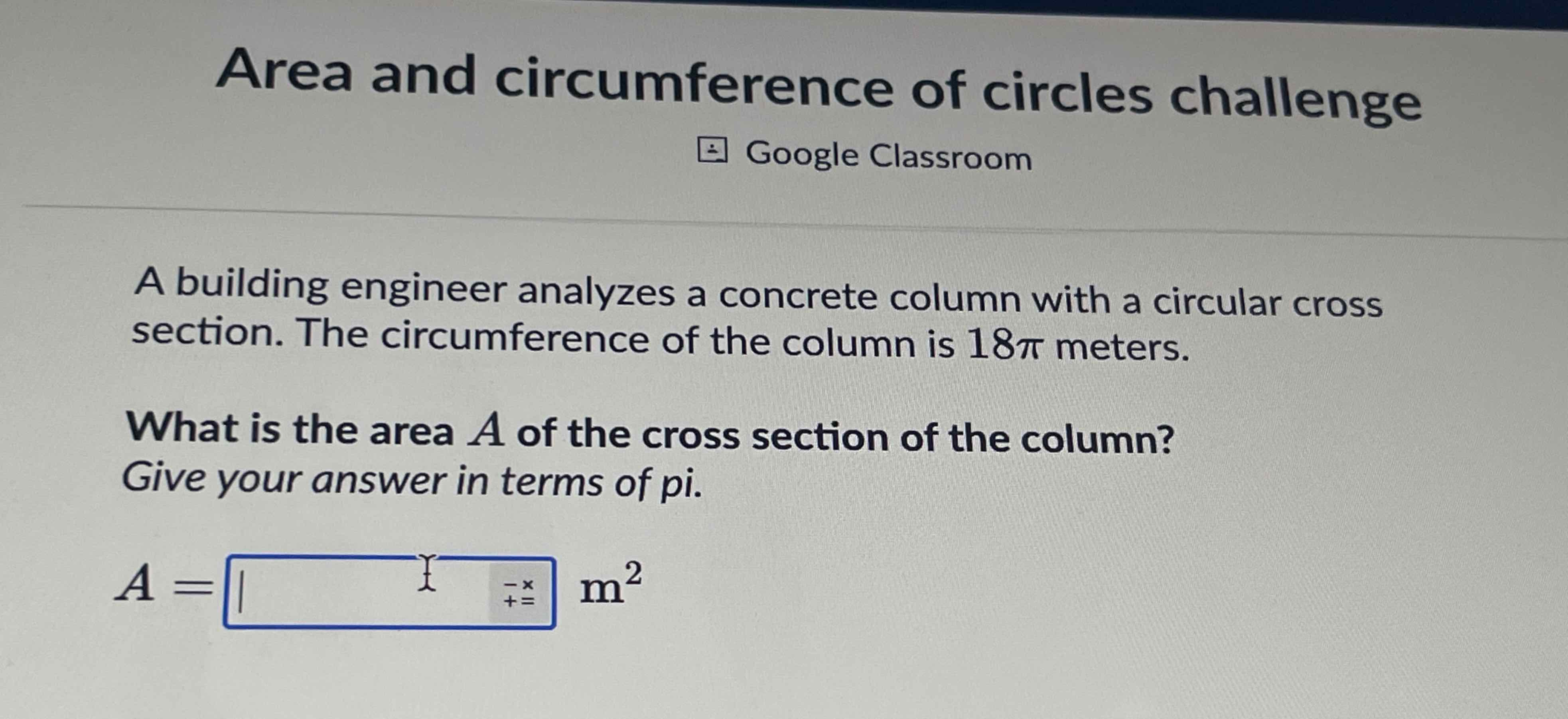
Q. A building engineer analyzes a concrete column with a circular cross section. The circumference of the column is . What is the area of the cross section of the column? Give your answer in terms of .
- Find Radius: Step : Find the radius of the circle using the circumference formula.Circumference formula: , where is the circumference and is the radius.Given meters.Set up the equation: .Solve for : meters.
- Calculate Area: Step : Calculate the area of the circle using the radius.Area formula: , where is the area and is the radius.Substitute meters into the formula: square meters.
More problems from Area of quadrilaterals and triangles: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help