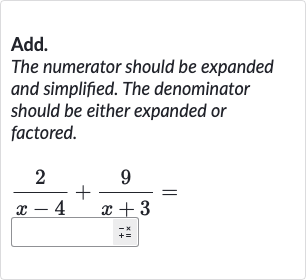
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (2)/(x-4)+(9)/(x+3)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (2)/(x-4)+(9)/(x+3)=

Bytelearn · Accepted Answer

Identify Denominators: Identify the denominators of the two fractions. The first fraction has a denominator of (x-4), and the second fraction has a denominator of (x+3).Find Common Denominator: Find a common denominator for the two fractions. The common denominator will be the product of the two distinct denominators, which is (x-4)(x+3).Rewrite with Common Denominator: Rewrite each fraction with the common denominator. The first fraction becomes (2)(x+3)/[(x-4)(x+3)], and the second fraction becomes (9)(x-4)/[(x-4)(x+3)].Expand Numerators: Expand the numerators of both fractions. For the first fraction, expand (2)(x+3) to get 2x + 6. For the second fraction, expand (9)(x-4) to get 9x - 36.Combine over Common Denominator: Combine the expanded numerators over the common denominator. The combined fraction is (2x + 6 + 9x - 36)/[(x-4)(x+3)].Simplify Numerator: Simplify the numerator of the combined fraction. Add the like terms in the numerator to get (2x + 9x) + (6 - 36), which simplifies to 11x - 30.Write Simplified Fraction: Write the simplified fraction. The simplified fraction is (11x - 30)/[(x-4)(x+3)].

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{2}{x-4}+\frac{9}{x+3}=$

Full solution

More problems from Negative Exponents

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline2x−4+9x+3= \frac{2}{x-4}+\frac{9}{x+3}= x−42​+x+39​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{2}{x-4}+\frac{9}{x+3}=$