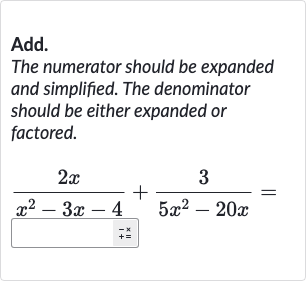
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (2x)/(x^(2)-3x-4)+(3)/(5x^(2)-20 x)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (2x)/(x^(2)-3x-4)+(3)/(5x^(2)-20 x)=

Bytelearn · Accepted Answer

Factor denominators: Factor the denominators of both fractions. We need to factor the denominators to find a common denominator for the addition. For the first fraction, the denominator is x^2 - 3x - 4, which factors into (x - 4)(x + 1). For the second fraction, the denominator is 5x^2 - 20x, which can be factored by taking out the common factor of 5x, resulting in 5x(x - 4).Identify LCD: Identify the least common denominator (LCD). The LCD for the fractions is the product of the distinct factors from both denominators, which is 5x(x - 4)(x + 1).Rewrite with LCD: Rewrite each fraction with the LCD as the new denominator. For the first fraction, we multiply the numerator and denominator by 5 to get (10x)/(5x(x - 4)(x + 1)). For the second fraction, we multiply the numerator and denominator by (x + 1) to get (3(x + 1))/(5x(x - 4)(x + 1)).Combine fractions: Combine the fractions over the common denominator. Now we add the two fractions together: (10x)/(5x(x - 4)(x + 1)) + (3(x + 1))/(5x(x - 4)(x + 1)) = (10x + 3(x + 1))/(5x(x - 4)(x + 1))Expand and simplify numerator: Expand and simplify the numerator. We distribute the 3 in the second term of the numerator: 10x + 3(x + 1) = 10x + 3x + 3 = 13x + 3Write final simplified expression: Write the final simplified expression. The final expression is: (13x + 3)/(5x(x - 4)(x + 1))

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{2 x}{x^{2}-3 x-4}+\frac{3}{5 x^{2}-20 x}=$

Full solution

More problems from Negative Exponents

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline2xx2−3x−4+35x2−20x= \frac{2 x}{x^{2}-3 x-4}+\frac{3}{5 x^{2}-20 x}= x2−3x−42x​+5x2−20x3​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{2 x}{x^{2}-3 x-4}+\frac{3}{5 x^{2}-20 x}=$