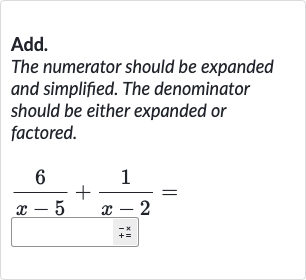
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (6)/(x-5)+(1)/(x-2)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (6)/(x-5)+(1)/(x-2)=

Bytelearn · Accepted Answer

Identify Common Denominator: Identify the common denominator for the fractions (6)/(x-5) and (1)/(x-2). To add fractions, we need a common denominator. The common denominator will be the product of the two distinct denominators (x-5) and (x-2). Common Denominator: (x-5)(x-2)Rewrite with Common Denominator: Rewrite each fraction with the common denominator. We need to adjust the numerators to reflect the new common denominator. (6)/(x-5) becomes (6)(x-2)/((x-5)(x-2)) (1)/(x-2) becomes (1)(x-5)/((x-5)(x-2))Expand Numerators: Expand the numerators of both fractions. Now we expand the numerators to simplify the expression. (6)(x-2) = 6x - 12 (1)(x-5) = x - 5Add Expanded Numerators: Add the expanded numerators together. Now we add the two expanded numerators while keeping the common denominator. (6x - 12) + (x - 5) = 7x - 17Write as Single Fraction: Write the sum as a single fraction. The sum of the two fractions with the common denominator is: (7x - 17)/((x-5)(x-2))

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{6}{x-5}+\frac{1}{x-2}=$

Full solution

More problems from Negative Exponents

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline6x−5+1x−2= \frac{6}{x-5}+\frac{1}{x-2}= x−56​+x−21​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{6}{x-5}+\frac{1}{x-2}=$