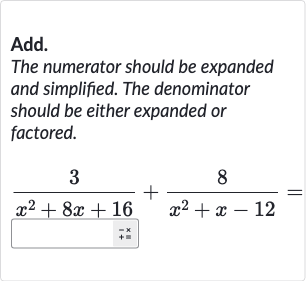
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (3)/(x^(2)+8x+16)+(8)/(x^(2)+x-12)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (3)/(x^(2)+8x+16)+(8)/(x^(2)+x-12)=

Bytelearn · Accepted Answer

Identify denominators: Identify the denominators of both fractions to determine if they can be factored. The first denominator is x^2 + 8x + 16, which is a perfect square trinomial and can be factored as (x + 4)(x + 4) or (x + 4)^2. The second denominator is x^2 + x - 12, which can be factored into (x + 4)(x - 3).Write fractions with factored denominators: Write the fractions with their factored denominators. (3)/(x^2 + 8x + 16) becomes (3)/((x + 4)^2). (8)/(x^2 + x - 12) becomes (8)/((x + 4)(x - 3)).Find common denominator: Find a common denominator for the two fractions. The least common denominator (LCD) is (x + 4)^2 * (x - 3).Rewrite fractions with common denominator: Rewrite each fraction with the common denominator. (3)/((x + 4)^2) becomes (3)(x - 3)/((x + 4)^2(x - 3)). (8)/((x + 4)(x - 3)) becomes (8)(x + 4)/((x + 4)(x - 3)(x + 4)).Combine fractions over common denominator: Combine the fractions over the common denominator. (3)(x - 3)/((x + 4)^2(x - 3)) + (8)(x + 4)/((x + 4)(x - 3)(x + 4)) becomes (3x - 9 + 8x + 32)/((x + 4)^2(x - 3)).Simplify numerator: Simplify the numerator by combining like terms. 3x - 9 + 8x + 32 simplifies to 11x + 23.Write final simplified fraction: Write the final simplified fraction. The sum of the fractions is (11x + 23)/((x + 4)^2(x - 3)).

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{3}{x^{2}+8 x+16}+\frac{8}{x^{2}+x-12}=$

Full solution

More problems from Powers with decimal and fractional bases

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline3x2+8x+16+8x2+x−12= \frac{3}{x^{2}+8 x+16}+\frac{8}{x^{2}+x-12}= x2+8x+163​+x2+x−128​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{3}{x^{2}+8 x+16}+\frac{8}{x^{2}+x-12}=$