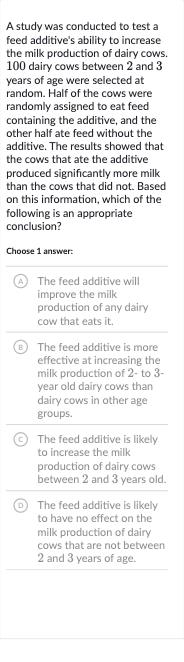
A study was conducted to test a feed additive's ability to increase the milk production of dairy cows. 100 dairy cows between 2 and 3 years of age were selected at random. Half of the cows were randomly assigned to eat feed containing the additive, and the other half ate feed without the additive. The results showed that the cows that ate the additive produced significantly more milk than the cows that did not. Based on this information, which of the following is an appropriate conclusion? Choose 1 answer: (A) The feed additive will improve the milk production of any dairy cow that eats it. (B) The feed additive is more effective at increasing the milk production of 2- to 3 year old dairy cows than dairy cows in other age groups. (C) The feed additive is likely to increase the milk production of dairy cows between 2 and 3 years old. (D) The feed additive is likely to have no effect on the milk production of dairy cows that are not between 2 and 3 years of age.

Question

Bytelearn · Accepted Answer

Study Design: The study involved 100 dairy cows between 2 and 3 years old, split into two groups of 50. One group was given feed with the additive, the other without.Results: The results showed a significant increase in milk production for the cows that ate the feed with the additive.Limitations: Since the study only included cows between 2 and 3 years old, we can't generalize the results to cows of other ages.Option (A): Option (A) is too broad because the study doesn't prove the additive will work for any cow.Option (B): Option (B) is incorrect because the study didn't compare the 2- to 3-year-old cows' milk production with that of cows in other age groups.Option (C): Option (C) is the most appropriate conclusion since the study showed a positive effect on the cows in the specified age group.Option (D): Option (D) is not supported by the study since it didn't include cows outside the 2- to 3-year-old age range.

Full solution

More problems from Interpret confidence intervals for population means

Related topics