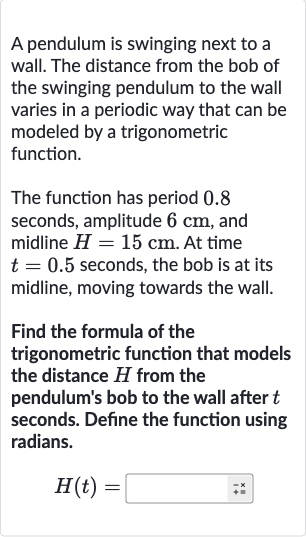
A pendulum is swinging next to a wall. The distance from the bob of the swinging pendulum to the wall varies in a periodic way that can be modeled by a trigonometric function. The function has period 0.8 seconds, amplitude 6cm, and midline H=15cm. At time t=0.5 seconds, the bob is at its midline, moving towards the wall. Find the formula of the trigonometric function that models the distance H from the pendulum's bob to the wall after t seconds. Define the function using radians. H(t)=◻_(◻)^(-×)

Question

A pendulum is swinging next to a wall. The distance from the bob of the swinging pendulum to the wall varies in a periodic way that can be modeled by a trigonometric function. The function has period 0.8 seconds, amplitude  6cm, and midline  H=15cm. At time  t=0.5 seconds, the bob is at its midline, moving towards the wall. Find the formula of the trigonometric function that models the distance  H from the pendulum's bob to the wall after  t seconds. Define the function using radians.  H(t)=◻_(◻)^(-×)

Bytelearn · Accepted Answer

Determine trigonometric function: Determine the trigonometric function to use. Since the pendulum is at its midline at t=0.5 seconds and moving towards the wall, we can use the cosine function, which has a value of 0 at π/2 radians (which corresponds to 0.5 seconds in this context). This is because the cosine function starts at its maximum value and decreases to 0 at π/2, which matches the description of the pendulum's motion.Calculate angular frequency: Calculate the angular frequency. The period T of the function is 0.8 seconds. The angular frequency ω is related to the period by the formula ω = 2π/T. Let's calculate ω. ω = 2π / 0.8 = 2π / (4/5) = (2π * 5) / 4 = (5π) / 2Write function with amplitude: Write the function with the amplitude and midline. The amplitude A is 6 cm, and the midline H is 15 cm. The general form of the cosine function is H(t) = A * cos(ωt) + H. Plugging in the amplitude and midline, we get: H(t) = 6 * cos((5π/2)t) + 15Adjust phase shift: Adjust the phase shift. Since the pendulum is at its midline at t=0.5 seconds, we need to introduce a phase shift to the function. The cosine function is at its midline when the argument of the cosine is π/2. We need to find the phase shift φ such that when t=0.5, the argument of the cosine function is π/2. Let's set up the equation: (5π/2) * 0.5 + φ = π/2 (5π/4) + φ = π/2 φ = π/2 - 5π/4 φ = -3π/4Write final function: Write the final function. Now we include the phase shift in the function: H(t) = 6 * cos((5π/2)t - 3π/4) + 15 This is the trigonometric function that models the distance from the pendulum's bob to the wall after t seconds.

Full solution

More problems from Scale drawings: word problems

Related topics