Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Which of the following radian measures is equal to 720^(@) ? (The number of degrees of arc in a circle is 360 . The number of radians of arc in a circle is 2pi.) Choose 1 answer: A pi radians (B) 2pi radians (c) 4pi radians (D) 8pi radians

Which of the following radian measures is equal to 720∘ 720^{\circ} ?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) π \pi radians\newline(B) 2π 2 \pi radians\newline(C) 4π 4 \pi radians\newline(D) 8π 8 \pi radians

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Convert between radians and degreesright chevron icon

Full solution

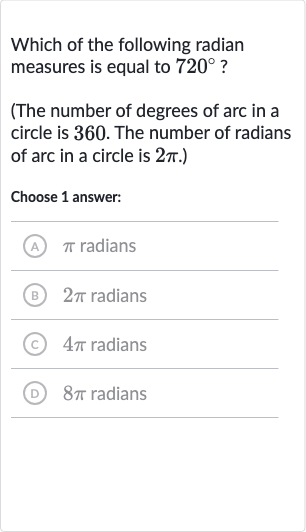
Q. Which of the following radian measures is equal to 720∘ 720^{\circ} ?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) π \pi radians\newline(B) 2π 2 \pi radians\newline(C) 4π 4 \pi radians\newline(D) 8π 8 \pi radians
  1. Understanding degrees and radians: Understand the relationship between degrees and radians. We know that 360360 degrees is equal to 2π2\pi radians. This means that 11 degree is equal to 2π360\frac{2\pi}{360} radians, which simplifies to π180\frac{\pi}{180} radians.
  2. Converting 720720 degrees to radians: Convert 720720 degrees into radians using the relationship from Step 11. Multiply 720720 degrees by the conversion factor π180\frac{\pi}{180} radians per degree.\newlineCalculation: 720720 degrees ×(π180 radians/degree)=4π\times \left(\frac{\pi}{180} \text{ radians/degree}\right) = 4\pi radians.
  3. Determining the radians for 720720 degrees: Choose the correct option of radians for 720720 degrees. According to the calculation in Step 22, 720720 degrees is equal to 4π4\pi radians.

More problems from Convert between radians and degrees

Question
Find the measure of a negative angle coterminal with 180∘180^\circ. \newline____∘^\circ
Get tutor helpright-arrow

Posted 3 months ago

Question
Find the measure of the reference angle for a 170∘170^\circ angle.
Get tutor helpright-arrow

Posted 2 months ago

Question
How many radians is 60∘60^\circ?\newlineChoices: \newline[A]π3 radians[A]\frac{\pi}{3}\text{ radians}\newline[B]π2 radians[B]\frac{\pi}{2}\text{ radians}\newline[C]π4 radians[C]\frac{\pi}{4}\text{ radians}\newline[D]π6 radians[D]\frac{\pi}{6}\text{ radians}
Get tutor helpright-arrow

Posted 3 months ago

Question
Which of the following radian measures is equal to 90∘ 90^{\circ} ?\newlineChoose 11 answer:\newline(A) π3 \frac{\pi}{3} radians\newlineB π4 \frac{\pi}{4} radians\newline(C) π2 \frac{\pi}{2} radians\newline(D) π6 \frac{\pi}{6} radians
Get tutor helpright-arrow

Posted 2 months ago

Question
Which of the following radian measures is equal to 225∘ 225^{\circ} ?\newlineChoose 11 answer:\newline(A) π4 \frac{\pi}{4} radians\newlineB π2 \frac{\pi}{2} radians\newline(C) 3π4 \frac{3 \pi}{4} radians\newline(D) 5π4 \frac{5 \pi}{4} radians
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following radian measures is equal to 135∘ 135^{\circ} ?\newlineChoose 11 answer:\newline(A) π4 \frac{\pi}{4} radians\newline(B) π2 \frac{\pi}{2} radians\newline(C) 3π4 \frac{3 \pi}{4} radians\newline(D) π \pi radians
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following radian measures is equal to 330∘ 330^{\circ} ?\newlineChoose 11 answer:\newline(A) 15π6 \frac{15 \pi}{6} radians\newlineB 7π6 \frac{7 \pi}{6} radians\newline(C) 11π6 \frac{11 \pi}{6} radians\newline(D) 13π6 \frac{13 \pi}{6} radians
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following radian measures is equal to 75∘ 75^{\circ} ?\newlineChoose 11 answer:\newline(A) π12 \frac{\pi}{12} radians\newlineB π3 \frac{\pi}{3} radians\newline(C) 5π12 \frac{5 \pi}{12} radians\newline(D) 7π12 \frac{7 \pi}{12} radians
Get tutor helpright-arrow

Posted 2 months ago

Question
Which of the following radian measures is equal to 240∘ 240^{\circ} ?\newlineChoose 11 answer:\newline(A) 4π3 \frac{4 \pi}{3} radians\newlineB 5π3 \frac{5 \pi}{3} radians\newline(C) 7π3 \frac{7 \pi}{3} radians\newline(D) 11π3 \frac{11 \pi}{3} radians
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following radian measures is equal to 120∘ 120^{\circ} ?\newlineChoose 11 answer:\newline(A) 2π3 \frac{2 \pi}{3} radians\newline(B) π \pi radians\newline(C) 4π3 \frac{4 \pi}{3} radians\newline(D) 5π3 \frac{5 \pi}{3} radians
Get tutor helpright-arrow

Posted 4 months ago

View all (43)