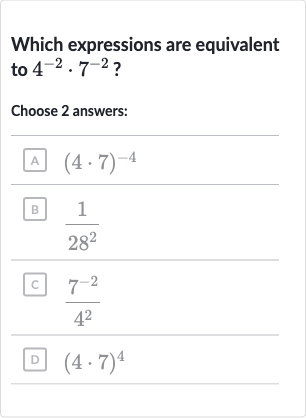
Which expressions are equivalent to 4^(-2)*7^(-2) ? Choose 2 answers: A (4*7)^(-4) B) (1)/(28^(2)) c) (7^(-2))/(4^(2)) D (4*7)^(4)

Question

Which expressions are equivalent to  4^(-2)*7^(-2) ? Choose 2 answers: A  (4*7)^(-4) B)  (1)/(28^(2)) c)  (7^(-2))/(4^(2)) D  (4*7)^(4)

Bytelearn · Accepted Answer

Understanding the expression: Understand the given expression and the properties of exponents. The given expression is 4^(-2)*7^(-2). According to the properties of exponents, when multiplying powers with the same exponent but different bases, you can combine the bases and keep the exponent.Combining the bases: Apply the property of exponents to combine the bases. 4^(-2)*7^(-2) can be written as (4*7)^(-2) because the exponents are the same. Calculation: (4*7)^(-2) = 28^(-2)Comparing the choices: Compare the result with the given choices. We have (4*7)^(-2) which is equivalent to 28^(-2). Now we need to find which choices match this expression.Analyzing choice A: Analyze choice A. Choice A is (4*7)^(-4). This is not equivalent to 28^(-2) because the exponent is -4, not -2.Analyzing choice B: Analyze choice B. Choice B is (1)/(28^(2)). This is equivalent to 28^(-2) because a negative exponent indicates the reciprocal of the base raised to the positive exponent. Calculation: 28^(-2) = 1/(28^2)Analyzing choice C: Analyze choice C. Choice C is (7^(-2))/(4^(2)). This is not equivalent to 28^(-2) because the bases are not multiplied together, and the exponents are not the same.Analyzing choice D: Analyze choice D. Choice D is (4*7)^(4). This is not equivalent to 28^(-2) because the exponent is 4, not -2, and it is positive, not negative.Concluding the correct choices: Conclude with the correct choices. The correct choices that are equivalent to 4^(-2)*7^(-2) are B) (1)/(28^(2)) and none of the other choices match.

Which expressions are equivalent to $\newline$ $4^{-2} \cdot 7^{-2}$ ? $\newline$ Choose $2$ answers: $\newline$ A) $\newline$ $(4 \cdot 7)^{-4}$ $\newline$ B) $\newline$ $\frac{1}{28^{2}}$ $\newline$ C) $\newline$ $\frac{7^{-2}}{4^{2}}$ $\newline$ D) $\newline$ $(4 \cdot 7)^{4}$

Full solution

More problems from Evaluate expressions using properties of exponents

Related topics