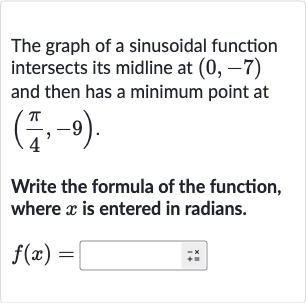
The graph of a sinusoidal function intersects its midline at (0,-7) and then has a minimum point at ((pi)/(4),-9). Write the formula of the function, where x is entered in radians. f(x)=

Question

The graph of a sinusoidal function intersects its midline at  (0,-7) and then has a minimum point at  ((pi)/(4),-9). Write the formula of the function, where  x is entered in radians.  f(x)=

Bytelearn · Accepted Answer

Determine Amplitude: Determine the amplitude of the function. The amplitude (A) is the distance from the midline to a maximum or minimum point on the graph. Since we have a minimum point at (-9) and the midline is at (-7), the amplitude is the absolute value of the difference between these two y-values. A = |-9 - (-7)| = |-9 + 7| = |2| = 2Determine Period: Determine the period of the function. Since we only have information about the function intersecting the midline and reaching a minimum, we cannot directly determine the period from the given information. However, we can assume that the function is a sine or cosine function, which typically have a period of 2π. We will use this assumption to proceed and adjust if necessary later.Determine Shifts: Determine the phase shift and vertical shift. The phase shift is the horizontal shift from the standard position of the sine or cosine function. Since the function intersects the midline at (0,-7), there is no horizontal shift, so the phase shift is 0. The vertical shift is the y-coordinate of the midline, which is -7.Identify Function Type: Determine whether the function is a sine or cosine function and its reflection. Since the function has a minimum point at ((pi)/(4),-9), and it intersects the midline at (0,-7) before reaching this minimum, we can conclude that it is a cosine function that has been reflected vertically (because the cosine function normally starts at a maximum). The reflection is indicated by a negative amplitude.Write Function Formula: Write the formula of the function. Using the information gathered, we can write the formula for the sinusoidal function. Since it's a reflected cosine function, the amplitude will be negative. There is no horizontal shift, so we don't need to include a phase shift in the formula. The vertical shift is -7. f(x) = -Acos(Bx - C) + D Where A is the amplitude, B is related to the period (B = 2π/period), C is the phase shift, and D is the vertical shift. Substituting the known values: A = 2 (but will be negative due to reflection) B = 1 (since we are assuming a standard period of 2π) C = 0 (no phase shift) D = -7 (vertical shift) f(x) = -2cos(x) - 7

The graph of a sinusoidal function intersects its midline at $(0,-7)$ and then has a minimum point at $\left(\frac{\pi}{4},-9\right)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=$

Full solution

More problems from Write exponential functions: word problems

Related topics

The graph of a sinusoidal function intersects its midline at (0,−7) (0,-7) (0,−7) and then has a minimum point at (π4,−9) \left(\frac{\pi}{4},-9\right) (4π​,−9).\newlineWrite the formula of the function, where x x x is entered in radians.\newlinef(x)= f(x)= f(x)=

The graph of a sinusoidal function intersects its midline at $(0,-7)$ and then has a minimum point at $\left(\frac{\pi}{4},-9\right)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=$