Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The functions
y=f(x) and
y=g(x) are graphed in the
xy-plane, where

f(x)=3x^(2)-3x+7" and "

g(x)=3x^(2)-3x-1". If the "
graph of function
f crosses the
y axis at
(0,b) and the graph of function
g crosses the
y-axis at
(0,c), then what is the value of
b-c ?

The functions $y=f(x)$ and $y=g(x)$ are graphed in the $x y$ -plane, where $\newline$ $f(x)=3 x^{2}-3 x+7 \text { and }$ $\newline$ $g(x)=3 x^{2}-3 x-1 \text {. If the }$ $\newline$ graph of function $f$ crosses the $y$ axis at $(0, b)$ and the graph of function $g$ crosses the $y$ -axis at $(0, c)$ , then what is the value of $b-c$ ?

View step-by-step help

View step-by-step help

Convert equations of parabolas from general to vertex form

Full solution

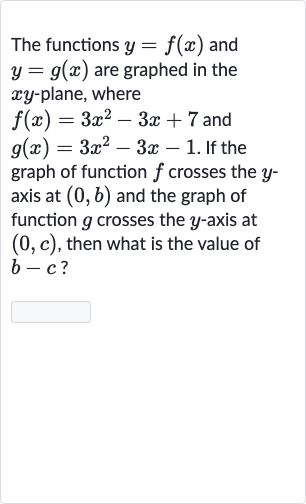
Q. The functions

y=f(x)

and

y=g(x)

are graphed in the

x y

-plane, where

\newline

f(x)=3 x^{2}-3 x+7 \text { and }

\newline

g(x)=3 x^{2}-3 x-1 \text {. If the }

\newline

graph of function

f

crosses the

y

axis at

(0, b)

and the graph of function

g

crosses the

y

-axis at

(0, c)

, then what is the value of

b-c

?

Identify y-intercepts: Identify the y-intercepts of the functions $f(x)$ and $g(x)$ . $\newline$ The y-intercept of a function occurs where $x = 0$ . To find the y-intercepts of $f(x)$ and $g(x)$ , we will substitute $x = 0$ into each function.
Calculate y-intercept of f(x): Calculate the y-intercept of f(x). Substitute $x = 0$ into $f(x) = 3x^2 - 3x + 7$ . $f(0) = 3(0)^2 - 3(0) + 7$ $f(0) = 0 - 0 + 7$ $f(0) = 7$ So, the y-intercept of f(x) is at $(0, 7)$ , which means $b = 7$ .
Calculate y-intercept of g(x): Calculate the y-intercept of g(x). $\newline$ Substitute $x = 0$ into $g(x) = 3x^2 - 3x - 1$ . $\newline$ $g(0) = 3(0)^2 - 3(0) - 1$ $\newline$ $g(0) = 0 - 0 - 1$ $\newline$ $g(0) = -1$ $\newline$ So, the y-intercept of g(x) is at $(0, -1)$ , which means $c = -1$ .
Calculate difference between y-intercepts: Calculate the difference between the y-intercepts $b$ and $c$ .
$b - c = 7 - (-1)$
$b - c = 7 + 1$
$b - c = 8$

More problems from Convert equations of parabolas from general to vertex form

Question

What is the focus of the parabola

y = -x^2

\newline

Simplify any fractions.

\newline

(_____ , _____)

\newline

Posted 2 months ago

Question

The equation of a parabola is

y = x^2 - 10x + 25

. Write the equation in vertex form.

\newline

Write any numbers as integers or simplified proper or improper fractions.

\newline

Posted 3 months ago

Question

In which direction does the parabola

x + y^2 = -7

\newline

\newline

\text{up}

\newline

\text{down}

\newline

\text{right}

\newline

\text{left}

Posted 3 months ago

Question

What is the vertex of the parabola

y = -4(x - 2)^2 + 2

?

\newline

(\_,\_)

Posted 3 months ago

Question

What is the axis of symmetry of the parabola

x = \frac{1}{2}(y + 3)^2 + 5

Posted 3 months ago

Question

In which direction does the parabola

x - 2y^2 = -5

\newline

\newline

\text{[A]up}

\newline

\text{[B]down}

\newline

\text{[C]right}

\newline

\text{[D]left}

Posted 3 months ago

Question

What is the vertex of the parabola

y - 8x^2 = 4

\newline

(\_,\_)

Posted 2 months ago

Question

The equation of a circle is

x^{2}+(y+4)^{2}=1

. What are the center and radius of the circle?

\newline

1

\newline

(A) The center is

(-4,0)

and the radius is

1

\newline

(B) The center is

(4,0)

and the radius is

1

\newline

(C) The center is

(0,4)

and the radius is

1

\newline

(D) The center is

(0,-4)

and the radius is

1

Posted 4 months ago

Question

y=(x-3)(x+9)

\newline

The given equation is graphed in the

x y

-plane. Which of the following are

x

-intercepts of the graph?

\newline

1

\newline

-3

-9

\newline

-3

9

\newline

3

-9

\newline

3

9

Posted 4 months ago

Question

y=2 x^{2}-5 x+7

is graphed in the

x y

-plane, which of the following characteristics of the graph is displayed as a constant or coefficient in the equation?

\newline

1

\newline

x

\newline

y

\newline

x

-coordinate of the vertex

\newline

y

-coordinate of the vertex

Posted 4 months ago