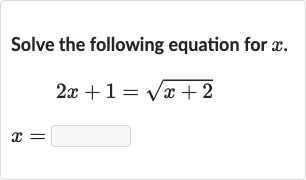
Solve the following equation for x. 2x+1=sqrt(x+2) x=

Question

Solve the following equation for  x.  2x+1=sqrt(x+2)  x=

Bytelearn · Accepted Answer

Square both sides: Square both sides of the equation to eliminate the square root. (2x + 1)^2 = (sqrt(x + 2))^2Expand using FOIL method: Expand the left side of the equation using the FOIL method (First, Outer, Inner, Last). (2x + 1)(2x + 1) = x + 2 4x^2 + 2x + 2x + 1 = x + 2Combine like terms: Combine like terms on the left side of the equation. 4x^2 + 4x + 1 = x + 2Subtract x from both sides: Subtract x from both sides of the equation to bring all x terms to one side. 4x^2 + 4x + 1 - x = x + 2 - x 4x^2 + 3x + 1 = 2Subtract 2 from both sides: Subtract 2 from both sides of the equation to set the equation to zero. 4x^2 + 3x + 1 - 2 = 0 4x^2 + 3x - 1 = 0Quadratic equation: Now we have a quadratic equation. We can either factor it, complete the square, or use the quadratic formula to solve for x. The quadratic formula is x = (-b ± sqrt(b^2 - 4ac)) / (2a). For this equation, a = 4, b = 3, and c = -1.Calculate discriminant: Calculate the discriminant (b^2 - 4ac) to determine if there are real solutions. Discriminant = 3^2 - 4(4)(-1) Discriminant = 9 - (-16) Discriminant = 9 + 16 Discriminant = 25Use quadratic formula: Since the discriminant is positive, there are two real solutions. Use the quadratic formula to find the values of x. x = (-3 ± sqrt(25)) / (2 * 4) x = (-3 ± 5) / 8Calculate possible values for x: Calculate the two possible values for x. x = (-3 + 5) / 8 or x = (-3 - 5) / 8 x = 2 / 8 or x = -8 / 8 x = 1/4 or x = -1Check solutions in original equation: We need to check both solutions in the original equation to ensure they do not result in taking the square root of a negative number. Check x = 1/4: 2(1/4) + 1 = sqrt(1/4 + 2) 1/2 + 1 = sqrt(2.25) 1.5 = 1.5 (True) Check x = -1: 2(-1) + 1 = sqrt(-1 + 2) -2 + 1 = sqrt(1) -1 = 1 (False)

Solve the following equation for $x$ . $\newline$ $2 x+1=\sqrt{x+2}$ $\newline$ $x=$

Full solution

More problems from Solve radical equations

Related topics

Solve the following equation for x x x.\newline2x+1=x+2 2 x+1=\sqrt{x+2} 2x+1=x+2​\newlinex= x= x=

Solve the following equation for $x$ . $\newline$ $2 x+1=\sqrt{x+2}$ $\newline$ $x=$