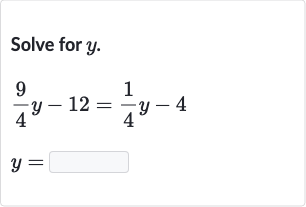AI tutor
Full solution
Q. Solve for .
- Isolate variable y: First, we need to isolate the variable y on one side of the equation. To do this, we will subtract from both sides to get all the y terms on one side.
- Simplify left side: Now, we simplify the left side by combining like terms.
- Rewrite equation: Since simplifies to , we rewrite the equation as:
- Isolate term with y: Next, we will add to both sides of the equation to isolate the term with .
- Solve for y: Finally, we divide both sides by to solve for y.
More problems from Solve linear equations with variables on both sides
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help