Full solution
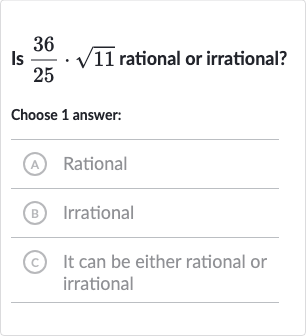
Q. Is rational or irrational?Choose answer:(A) Rational(B) Irrational(C) It can be either rational or irrational
- Evaluate expression: Evaluate the expression . is a rational number because it can be expressed as a fraction of two integers. However, is an irrational number because it cannot be expressed as a fraction of two integers; the square root of a non-perfect square is always irrational.
- Nature of product: Determine the nature of the product of a rational number and an irrational number.The product of a rational number and an irrational number is always irrational.This is because if the product were rational, then you could express the irrational number as the quotient of the rational product and the rational number, which is not possible.
- Apply rule: Apply the rule to the given expression.Since is rational and is irrational, their product must be irrational.
More problems from Classify rational and irrational numbers
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help