Full solution
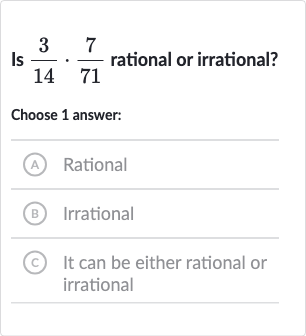
Q. Is rational or irrational?Choose answer:(A) Rational(B) Irrational(C) It can be either rational or irrational
- Define Rational Number: Define what a rational number is.A rational number is a number that can be expressed as the quotient or fraction of two integers, where and are integers and is not zero.
- Analyze Given Expression: Analyze the given expression.The given expression is . Both and are integers, and both and are integers that are not zero.
- Multiply Numerators and Denominators: Multiply the numerators and denominators.
- Check Result for Rational Number: Check if the result is a rational number.Since and are both integers and is not zero, the fraction represents a rational number.
- Answer Question Prompt: Answer the question prompt.The expression is a rational number because it can be expressed as a fraction of two integers with a non-zero denominator.
More problems from Classify rational and irrational numbers
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help