Full solution
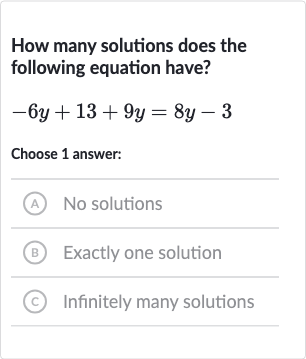
Q. How many solutions does the following equation have?Choose answer:(A) No solutions(B) Exactly one solution(C) Infinitely many solutions
- Combine like terms: Combine like terms on the left side of the equation.
- Rewrite equation: Rewrite the equation with the simplified left side.
- Subtract y terms: Subtract from both sides to get all the y terms on one side.
- Add to isolate y: Add to both sides to isolate the term.
- Divide to solve for y: Divide both sides by to solve for y.
- One solution: Since we found a specific value for , the equation has exactly one solution.
More problems from Find the number of solutions to a linear equation
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help