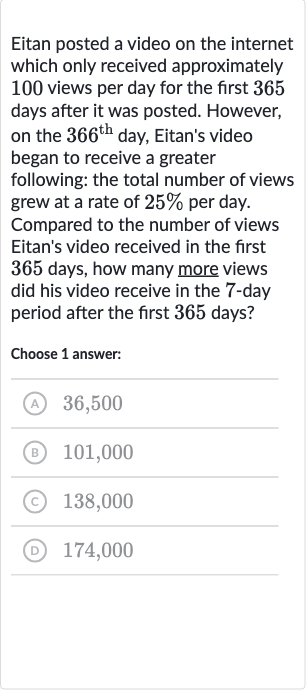
Eitan posted a video on the internet which only received approximately $100$ views per day for the first $365$ days after it was posted. However, on the $366^{\text {th }}$ day, Eitan's video began to receive a greater following: the total number of views grew at a rate of $25 \%$ per day. Compared to the number of views Eitan's video received in the first $365$ days, how many more views did his video receive in the $7$ -day period after the first $365$ days? $\newline$ Choose $1$ answer: $\newline$ (A) $36$ , $500$ $\newline$ (B) $101$ , $000$ $\newline$ (C) $138$ , $000$ $\newline$ (D) $174$ , $000$

View step-by-step help

Home

Math Problems

Algebra 1

Multi-step problems with unit conversions

Full solution

Q. Eitan posted a video on the internet which only received approximately

100

views per day for the first

365

days after it was posted. However, on the

366^{\text {th }}

day, Eitan's video began to receive a greater following: the total number of views grew at a rate of

25 \%

per day. Compared to the number of views Eitan's video received in the first

365

days, how many more views did his video receive in the

7

-day period after the first

365

days?

\newline

Choose

1

answer:

\newline

(A)

36

500

\newline

(B)

101

000

\newline

(C)

138

000

\newline

(D)

174

000

Calculate total views for first $365$ days: First, let's rephrase the ＂How many more views did Eitan's video receive in the $7$ -day period after the first $365$ days compared to the first $365$ days?＂
Calculate views on the $366$ th day: Calculate the total number of views for the first $365$ days. $\newline$ $100 \text{ views/day} \times 365 \text{ days} = 36,500 \text{ views}$
Calculate sum of views for $7$ days after the $365$ th day: Now, let's calculate the number of views on the $366$ th day. $\newline$ Since the views grew by $25\%$ from the original $100$ views per day, the views on the $366$ th day would be: $\newline$ $100$ views + $(\frac{25}{100} \times 100$ views) = $125$ views
Evaluate the sum: For the subsequent days, the number of views will increase by $25\%$ each day. This is a geometric sequence where the first term is $125$ views and the common ratio is $1.25$ (since $25\%$ increase is the same as multiplying by $1.25$ ). We need to find the sum of views for $7$ days starting from the $366$ th day. $\newline$ The sum of a geometric series is given by $S_n = a \cdot (1 - r^n) / (1 - r)$ , where $a$ is the first term, $r$ is the common ratio, and $125$ $0$ is the number of terms. $\newline$ In this case, $125$ $1$ , $125$ $2$ , and $125$ $3$ .
Calculate more views in the $7$ -day period after the first $365$ days: Calculate the sum of the geometric series for the $7$ days after the $365$ th day. $\newline$ $S_7 =$ $125$ \times ( $1$ - $1$ . $25$ ^ $7$ ) / ( $1$ - $1$ . $25$ )
Calculate more views in the $7$ -day period after the first $365$ days: Calculate the sum of the geometric series for the $7$ days after the $365$ th day. $\newline$ $S_7 = 125 \times (1 - 1.25^7) / (1 - 1.25)$ Evaluate the sum $S_7$ . $\newline$ $S_7 = 125 \times (1 - 1.25^7) / (1 - 1.25)$ $\newline$ $S_7 = 125 \times (1 - 17.37890625) / (-0.25)$ $\newline$ $S_7 = 125 \times (-16.37890625) / (-0.25)$ $\newline$ $S_7 = 125 \times 65.515625$ $\newline$ $S_7 = 8,189.453125$ $\newline$ Since we are looking for an integer number of views, we can round this to $8,189$ views.
Calculate more views in the $7$ -day period after the first $365$ days: Calculate the sum of the geometric series for the $7$ days after the $365$ th day. $\newline$ $S_7 = 125 \times (1 - 1.25^7) / (1 - 1.25)$ Evaluate the sum $S_7$ . $\newline$ $S_7 = 125 \times (1 - 1.25^7) / (1 - 1.25)$ $\newline$ $S_7 = 125 \times (1 - 17.37890625) / (-0.25)$ $\newline$ $S_7 = 125 \times (-16.37890625) / (-0.25)$ $\newline$ $S_7 = 125 \times 65.515625$ $\newline$ $S_7 = 8,189.453125$ $\newline$ Since we are looking for an integer number of views, we can round this to $8,189$ views.Now, we need to find out how many more views the video received in the $7$ -day period after the first $365$ days compared to the first $365$ days. $\newline$ More views = $S_7 - 36,500$ $\newline$ More views = $8,189 - 36,500$ $\newline$ This calculation is incorrect because we should be adding the views from the $7$ -day period to the initial $S_7$ $0$ views, not subtracting. This is a math error.