Full solution
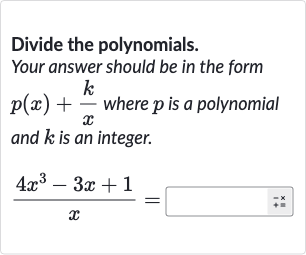
Q. Divide the polynomials.Your answer should be in the form where is a polynomial and is an integer.
- Divide by x individually: Divide each term of the polynomial by x individually.Divide by , by , and by . remains as it is because is not divisible by .
- Combine the division results: Combine the results of the division.The polynomial part is the combination of the terms that were divided without a remainder.)The remainder is the term that could not be divided evenly by \$ x \), which is \( \frac{1}{x} \).
- Write the final answer: Write the final answer in the form \(p(x) + \frac{k}{x}\).\(\newline\)The polynomial part is \(4x^2 - 3\), and the remainder is \(\frac{1}{x}\).\(\newline\)So, the final answer is \(4x^2 - 3 + \frac{1}{x}\).